The following are some of the multiple questions from the recent January 2019 New York State Common Core Geometry Regents exam.
Each correct answer is worth up to 2 credits. No partial credit. Work need not be shown.
1. After a dilation with center (0,0), the image of DB is D'B'.
If DB = 4.5 and D'B' = 18, the scale factor of this dilation is
Answer: (4) 4
2. In the diagram below, triangle ABC with sides of 13, 15, and 16, is mapped onto triangle DEF after a clockwise rotation of 90° about point P.
Answer: (4)8.5
3. On the set of axes below, triangle ABC has vertices at A(2,0), B(2,4), C(4,2), and triangle DEF has vertices at D(4,0), E(4,8), F(8,4).
Answer: (3) a dilation of ABC by a scale factor of 2 centered at the origin,
followed by a rotation of 180° about the origin
4. The figure below shows a rhombus with noncongruent diagonals.
Answer: (3) a clockwise rotation of 90° about the intersection of the
diagonals
5. In the diagram below of circle O, points K, A, T, I, and E are on the
circle, triangle KAE and triangle ITE are drawn, arc KE = arc EI, and ∠EKA = ∠EIT.
Answer: (4) They are congruent.
6. In right triangle ABC shown below, point D is on AB and point E is
on CB such that AC || DE.
Answer: (2) 6.25
7. In rhombus VENU, diagonals VN and EU intersect at S.
If VN = 12 and EU = 16, what is the perimeter of the rhombus?
Answer: (2) 40
8. Given right triangle ABC with a right angle at C, m∠B = 61°. Given right triangle RST with a right angle at T, m∠R = 29°.
Answer: (1) AB/RS = RT/AC
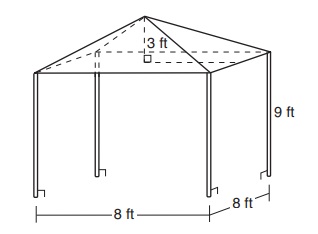
9. A vendor is using an 8-ft by 8-ft tent for a craft fair. The legs of the
tent are 9 ft tall and the top forms a square pyramid with a height of
3 ft.
Answer: (2) 640
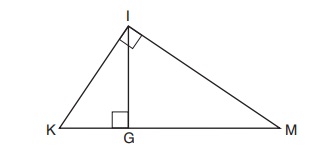
10. In the diagram below of right triangle KMI, altitude IG is drawn to
hypotenuse KM.
Answer: (3) 20
The other way to do this: The Right Triangle Altitude Theorem tells us that KG/IG = IG/GM.
11. Which three-dimensional figure will result when a rectangle 6 inches
long and 5 inches wide is continuously rotated about the longer side?
Answer: (3) a cylinder with a radius of 5 inches and a height of 6 inches
12. Which statement about parallelograms is always true?
Answer: (2) The diagonals bisect each other.
13. From a point on the ground one-half mile from the base of a historic
monument, the angle of elevation to its top is 11.87°. To the nearest foot, what is the height of the monument?
Answer: (2) 555
14. 4 The area of a sector of a circle with a radius measuring 15 cm is
75π cm2. What is the measure of the central angle that forms the sector?
Answer: (2) 120°
15. 5 Point M divides AB so that AM:MB = 1:2. If A has coordinates
(-1,-3) and B has coordinates (8,9), the coordinates of M are
Answer: (1) (2,1)
16. In the diagram below of triangle ABC, AC is extended through
point C to point D, and BE is drawn to AC.
Answer: (4) m∠7 = m∠3 + m∠2
The Exterior Angle Theorem states that the exterior angle of a triangle equals the sum of the two remote angles. This is because ∠2 + ∠3 + ∠4 = 180, because a triangle has 180 degrees, and ∠7 + ∠4 = 180, because supplementary angles add up to 180 degrees. So ∠2 + ∠3 + ∠4 = ∠7 + ∠4 and therefore ∠2 + ∠3 = ∠7.
17. In the diagram below of right triangle ABC, AC = 8, and AB = 17.
Answer: (4) tan A = 15/8
18. Francisco needs the three pieces of glass shown below to complete
a stained glass window. The shapes, two triangles and a trapezoid,
are measured in inches.
Answer: (1) 20
19. In the diagram of quadrilateral NAVY below, m∠YNA = 30°,
m∠YAN = 38°, m∠AVY = 94°, and m∠VAY = 46
Answer: (3) VA
20. What is an equation of a circle whose center is (1,4) and diameter is
10?
Answer: (1) x2 - 2x + y2 - 8y = 8
21. On the set of axes below, ABC, altitude , and median are
drawn.
Answer: (4) (GC)(AB) / 2
22. In right triangle ABC, m∠C = 90° and AC != BC. Which trigonometric ratio is equivalent to sin B?
Answer: (1) cos A
23. As shown in the diagram below, the radius of a cone is 2.5 cm and
its slant height is 6.5 cm.
Answer: (1) 12.5π
Volume of a cone is 1/3 * r2 * h * π = (1/3)(2.5)2(6) * π = 12.5π
Common mistakes: using the slant height as the height, and forgetting the (1/3). Both of those are incorrect choices.
24. LWhat is an equation of the image of the line y = (3/2)x - 4 after a
dilation of a scale factor of 3/4 centered at the origin?
Answer: (4) y = (3/2)x - 3
End of Part I
How did you do?
Questions, comments and corrections welcome.
More Geometry problems.
If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.
Thank you.
The answers to Part II can be found here
The answers to Parts III and IV can be found here
January 2019 Geometry, Part I
18 / 4.5 = 4. D'B' is four times bigger than DB.
If DE = 2x - 1, what is the value of x?
In a rotation, size is preserved. Side AB maps on side DE, so 2x = 1 = 16
2x = 17
x = 8.5
Which sequence of transformations will map ABC onto DEF?
ABC is the smaller triangle. To map it onto DEF, you need a scale factor of 2, not 1/2, which eliminates (2) and (4). However, just doubling the size will not adjust the orientation, so (1) is out.
Which transformation would not carry this rhombus onto itself?
If you rotate 90 degrees, the horizontal lines become vertical lines. The rhombus doesn't have vertical lines, so it isn't going to map onto itself.
Which statement about triangle KAE and triangle ITE is always true?
Arc KE = arc EI gives us two pieces of information: Chords KE and EI are congruent, and ∠A and ∠T are congruent. We are given ∠EKA = ∠EIT. By AAS, the triangles are congruent. We have no information that they are right triangles (they could be), but this choice can logically be eliminated. Even if they were right triangles, one of the other three choices would have to be true!
If AB = 15, BC = 12, and EC = 7, what is the length of BD?
The triangles are proportional in size, but we need to know the length of side BE.
If EC = 7, then BE = 12 - 7 = 5.
AB / BC = BD / BE
15 / 12 = BD / 5
12 BD = (15)(5)
12 BD = 75
BD = 6.25
If the diagonals have lengths 12 and 16 and they bisect each other, then the rhombus can be divided into four right triangles with legs of 6 and 8. That makes the hypotenuses of those triangles equal to 10, which is the length of one side of the rhombus. So the perimeter is 40.
Which proportion in relation to triangle ABC and triangle RST is not correct?
Angle A = Angle R, Angle B = Angle S. AB corresponds to RS, BC corresponds to ST, and AC corresponds to RT.
In Choice (1), the second ratio is upside down. It goes big to small equals small to big.
What is the volume, in cubic feet, of space the tent occupies?
The bottom of the tent is a prism. L * W * H = 8 * 8 * 9 = 576 (Eliminate Choice (1).)
The top of the tent is a prism. 1/3 * L * W * H = 1/3 * 8 * 8 * 3 = 64.
Total is 640.
If KG = 9 and IG = 12, the length of IM is
Hopefully, you realized right away that triangle IGK is a Pythagorean Triple, 9-12-15, a multiple of the 3-4-5 right triangle.
Also triangles IGK, IGM and IKM are all similar, so each of them is proportional and a multiple of 3-4-5.
IK is the hypotenuse of the IGK, and the shorter leg of IKM. Triangle IKM is 15-4n-5n. Since 15 = 3 times 5, the other two legs are 4 * 5 = 20 and 5 * 5 = 25. So IM is 20.
So (KG)(GM) = (IG)2
(9)(GM) = (12)2
(9)(GM) = 144
GM = 16.
IG = 12, GM = 16. By Pythagorean Theorem (or recognizing 3-4-5), IM = 20.
Rotating a rectangle about an axis will result in a cylinder because the rectangle is traveling a circular path. Eliminate (1) and (2).
If it's rotating about the longer side than that side will be the height. The side moving is a circle will be the radius.
This is true for all parallelograms. Choice (1) is only true for rectangles. Choice (3) is only true for rhombuses. Choice (4) is only true for squares.
One-half mile is (1/2)(5280) = 2640 feet
You have adjacent (the distance) and you need the opposite (the height). So use Tangent.
tan 11.87° = x / 2640
x = 2640 tan 11.87° = 554.89...
A = π r2 = π(15)2 = 225π.
225π / 75π = 360 / x. (At this point, drop the π, because they factor out.)
225x = 75 * 360
225x = 27000
x = 120
The ratio 1:2 means that point M is 1/3 of the distance away from point A and 2/3 of the distance from point B.
Find 1/3 of the difference of the x-coordinates and 1/3 of the difference of the y-coordinates.
(8 - -1)/3 = 9/3 = 3. -1 + 3 = 2, so the x-coordinate of M is 2. The answer must be Choice (1).
(9 - -3)/3 = 12/3 = 4. -3 + 4 = 1, so the y-coordinate of M is 1.
Which equation is always true?
Which equation would determine the value of angle A?
You have the adjacent side and the hypotenuse, so the easiest way to find A would be cos A = 8/17. However, that isn't one of the choices, so we need to find something else.
Notice that 15 is in the choices. Where does this come from? It's the missing leg of the triangle.
Again, this is a common Pythagorean Triple: 8-15-17. However, if you didn't notice this, you can figure it out:
172 - 82 = 289 - 64 = 225 = 152
Now look at the choices:
Choice (1) uses the adjacent side instead of the opposite.
Choice (2) has the adjacent over the opposite, instead of the other way around.
Choice (3) has the opposite side instead of the adjacent.
Choice (4) has opposite over adjacent, which is correct.
Glass can be purchased in rectangular sheets that are 12 inches wide.
What is the minimum length of a sheet of glass, in inches, that
Francisco must purchase in order to have enough to complete the
window?
The two smaller triangles can be placed adjacent to the trapezoid, matching up the side lengths, to create a rectangle that is 12 x 20 inches.
Which segment has the shortest length?
Angle V is 94 and angle VAY = 46, so angle AYV = 180 - (94 + 46) = 30. The smallest side of triangle VAY is the short across from the shortest angle, which would be VA.
Start with the standard form of the equation:
(x - h)2 + (y - k)2 = r2
(x - 1)2 + (y - 4)2 = 52 (Diameter is 10. Radius is 5.)
x2 - 2x + 1 + y2 - 8y + 16 = 25 (Eliminate Choices (2) and (4).)
x2 - 2x + y2 - 8y = 25 - 16 - 1 = 8 Choice (1)
Which expression represents the area of triangle ABC?
The area of a triangle is 1/2 times base times altitude. Notice I said "altitude" and not "height". To get an altitude, we need a right angle. You can tell a right angle on a graph by perpendicular lines, which have negative reciprocal slopes.
MC has a slope of -1. None of the sides have a slope of 1.
GC has a slope of -1/2. Side AB has a slope of 2, which is the negative reciprocal. So AB is the base and GC is an altitude.
Since AC is not equal to BC, the angles cannot be 45 degrees each, which would mean there were two possible answers.
Sin B is always the same as cos A.
This is because the side opposite B is adjacent to A.
How many cubic centimeters are in the volume of the cone?
You're given the slant height, but you need the height. The slant height forms a right triangle with the height and the radius.
So h = (6.52 - 2.52)(1/2) = 6.
Hint: if you look at the answers, not of the multiples of pi are irrational, meaning that the sides of the right triangle form a Pythagorean Triple. If you double the numbers given to get rid of the decimals, we have 5/?/13. There's a 5-12-13 right triangle. Half of 12 is 6.
If you didn't see that, you still have Pythagorean Theorem and a calculator.
A dilation would not change the slope of a line, so eliminate Choices (1) and (2).
A dilation would only change the y-intercept UNLESS the line goes through the center of dilation. The center of dilation is (0, 0) but the line goes through (0, -4), the y-intercept. Therefore, choice (3) is not correct.
By elimination, you know that the answer is (4).
Also, the y-intercept would have to be 3/4 of the former value. (3/4)(-4) = -3.
I also write Fiction!
Check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides.
Available in softcover or ebook at Amazon.

Wednesday, April 10, 2019
January 2019 Common Core Geometry Regents, Part I (multiple choice)
Labels:
Common Core,
Geometry,
Regents,
testing
Subscribe to:
Post Comments (Atom)




























No comments:
Post a Comment