The following are some of the multiple questions from the August 2019 New York State Geometry Regents exam.
Each correct answer is worth up to 4 credits. Partial credit is available. Work must be shown. Correct answers without work receive only 1 point.
32. Triangle ABC is shown below. Using a compass and straightedge, construct the dilation of Triangle ABC centered at B with a scale factor of 2.
[Leave all construction marks.]
Is the image of triangle ABC similar to the original triangle? Explain why.
Answer:
The image is similar to the original triangle because a dilation preserves the shape of the object. Therefore, the angles are the same size and by the AA Theorem, the two triangles are similar.
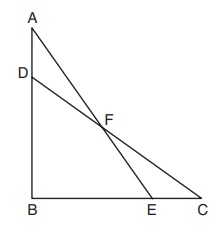
33. In the diagram below, triangle ABE = triangle CBD.
Prove: triangle AFD = triangle CFE
Answer:
You don't know that AD = CE directly, but you do know that AB = CB and DB = EB because of the congruency of the larger triangles. Therefore if you subtract the smaller segment from the larger one, you get the sides that we need to be congruent.
Your proof would go something like this:
34. A cargo trailer, pictured below, can be modeled by a rectangular prism and a triangular prism.
Inside the trailer, the rectangular prism measures 6 feet wide and 10 feet long. The walls that
form the triangular prism each measure 4 feet wide inside the trailer. The diagram below is of
the floor, showing the inside measurements of the trailer.
Answer:
32 + x2 = 42
A correct answer is worth up to 6 credits. Partial credit is available.
35. The coordinates of the vertices of Triangle ABC are A(1,2), B(-5,3), and C(-6,-3).
Prove that Triangle ABC is isosceles
State the coordinates of point D such that quadrilateral ABCD is a square.
Answer:
The second and third parts are really the same question. Once you have the square, you have to prove that it is a square. This is more rigorous than just an "explanation" or "justification". However, by this point you have already shown that two consecutive sides are congruent, so all you have to do is show that the sides are parallel, making it both a parallelogram and a rhombus, and that there is at least one right angle, which makes it a square.
First Part:
Second Part:
Third part:
AB || CD, AD || BC because they have the same slopes.
A quadrilateral with opposite sides parallel, with consecutive sides equal and a right angle must be a square.
End of Exam
How did you do?
August 2019 Geometry, Part III
Starting at point B, open the compass to Point A. Then from point A, make an arc. Use the straightedge to extend BA to the new arc. This line will have twice the length of BA.
Repeat the process for BC.
Finally, using the straightedge, complete the triangle from the endpoints of the two new line segments you constructed.
You need to prove that those two tiny triangles are similar.
You can see that Angles A and C are equal from the original triangles. And angles AFD and CFE are vertical angles. You need to have one pair of congruent sides.
Statement Reason 1. Triangle ABE = Triangle CBD 1. Given 2. Angle A = Angle C 2. CPCTC 3. Angle AFD = Angle CFE 3. Vertical angles are congruent. 4. AB = CB; DB = EB 4. CPCTC 5. AD = CE 5. Subtraction Postulate 6. triangle AFD = triangle CFE/td> 6. AAS
If the inside height of the trailer is 6.5 feet, what is the total volume of the inside of the trailer, to the nearest cubic foot?
Volume = Area of the Base times the Height.
The base is shaped like a pentagon, which, in this case, can be broken into a rectangle and a triangle. We have the base of the triangle, 6 feet; however, we need to solve for its height (lower case h). We can use the Pythagorean Theorem for this.
9 + x2 = 16
x2 = 7
x = SQRT(7) = 2.65 (approximately)
Area of the base = (6)(10) + (1/2)(6)(2.65) = 67.95
Volume = Area * height = 67.95 * 6.5 = 441.675 = 442 cubic feet
Part IV
[The use of the set of axes on the next page is optional.]
Prove that your quadrilateral ABCD is a square.
[The use of the set of axes below is optional.]
Two separate questions.
First, to prove that ABC is isosceles, you will have to show that two of the sides have the same length.
It can be assumed from the second part of the question even before we start that it will be a right triangle, because that is the only way that you will be able to add one more point and have a square. Furthermore, AB and BC would have to be the congruent sides.
Length of AB = SQRT( (-5 - 1)2 + (3 - 2)2 ) = SQRT(37)
Length of BC = SQRT( (-6 - -5)2 + (-3 - 3)2 ) = SQRT(37)
AB = BC, so triangle ABC is isosceles.
(No reason to check the length of AC.
To get from point A to B, you have to go down 6 left and up 1. (This can be seen if you graph it, or if you worked out the math above.) CD would have to have the same slope, but to get from C to D, you would have to go 6 right and down 1. So D would have to be (-6 + 6, -3 - 1) or D(0, -4).
The slope of AB = -1/6. The slope of CD = -1/6
The slope of BC = (-3 -3)/(-6 - -5) = -6/-1 = 6
The slope of DA = (-4 - 2)/(0 - 1) = -6 / -1 = 6
AB is perpendicular to BC because their slopes are inverse reciprocals (have a product of -1).
So Angle B is a right angle.
Comments and corrections welcome. (I get many of the latter!)
Friday, August 30, 2019
August 2019 Geometry Regents Parts 3 & 4
Thursday, August 29, 2019
Numberpalooza
(C)Copyright 2019, C. Burke.
Apparently, many (x, why?) songs are sung by shapes or symbols. And most of my Zero characters were *not* singers.
I thought it was going to be a few zeroes and tens when I started going through the old comics. And I never did, say, Boy Four-ge, even though a song was used for a truth table.
Come back often for more funny math and geeky comics.
Wednesday, August 28, 2019
August 2019 Geometry Regents, Part 2
The following are some of the multiple questions from the recent August 2019 New York State Common Core Geometry Regents exam.
Each correct answer is worth up to 2 credits. Partial credit can be given. Work must be shown or explained.
25.
In parallelogram ABCD shown below, m∠DAC = 98° and m∠ACD = 36°.
Answer:
26. An airplane took off at a constant angle of elevation. After the plane traveled for 25 miles,
it reached an altitude of 5 miles, as modeled below.
Answer:
27. On the set of axes below, Triangle ABC = Triangle DEF.
Answer:
A 180 degree rotation of ABC about the origin, followed by a translation of 4 spaces up.
A reflection of ABC across the y-axis, followed by a reflection across the line y = 2.
A reflection of ABC across the y-axis, followed by a reflection across the x-axis, followed by a translation 4 spaces up.
A 180 degree rotation of ABC about the point (0, 2).
You could also pick any point A, B, or C, and translate ABC so that it moves the point onto the corresponding point of DEF, and then rotate 180 degrees about that corresponding point. (You need to fill in the specifics -- the previous sentence by itself will not give you credit.)
28.
The vertices of Triangle ABC have coordinates A(-2,-1), B(10,-1), and C(4,4). Determine and state
the area of Triangle ABC. [The use of the set of axes below is optional.]
Answer:
b = 10 - (-2) = 12
Note that 72/360 is 1/5, which after a year of Geometry, you should probably recognize. One-fifth of ten squared is 1/5 of 100, which is 20.
29.
Using the construction below, state the degree measure of ∠CAD. Explain why.
Answer:
Triangle ABC is an equilateral triangle because sides AC and BC are constructed to be the same length of AB.
Note: You could have used a less wordy explanation than that, but I like to explain.
30.
In the diagram below of circle K, secant PLKE and tangent PZ are drawn from external point P.
Answer:
The measure of angle P can be found by taking half of the difference between arc EZ and LZ:
Alternatively, because arc LZ measures 56 degrees, then central angle LKZ has a measure of 56 degrees. If you draw radius KZ, it will be perpendicular to tangent PZ. That means that PKZ is a right triangle and you already know the size of two of its angles.
31.
A large water basin is in the shape of a right cylinder. The inside of the basin has a diameter of 8 1/4 feet and a height of 3 feet. Determine and state, to the nearest cubic foot, the number of
cubic feet of water that it will take to fill the basin to a level of 1/2 foot from the top.
Answer:
End of Part II
How did you do?
Questions, comments and corrections welcome.
August 2019 Geometry, Part II
What is the measure of angle B? Explain why.
The opposite angles in a parallelogram are congruent, so m∠B = m∠D. Also, ACD is a triangle, so the sum of its angles is 180 degrees.
180 - 98 - 36 = 46 degrees.
Angle D is 46 degrees, so angle B is 46 degrees.
To the nearest tenth of a degree, what was the angle of elevation?
The angle of elevation is measured from the ground. That makes the altitude of 5 miles the side opposite of the angle, and the 25 miles is the hypotenuse. This means you need to use sine.
Sin x = 5/25
x = sin-1 (1/5) = 11.53...
The angle of elevation is 11.5 degrees, to the nearest tenth.
Describe a sequence of rigid motions that maps Triangle ABC onto Triangle DEF.
Some possibilities
Since A and B both have a y-coordinate of -1, you know that AB is a horizontal line and you can use that as the base of your triangle. The height of the triangle is the vertical distance from -1 to 4. And the area of the triangle is 1/2 (base) (height).
h = 4 - (-1) = 5
A = 1/2(12)(5) = 30
A reverse construction question! I like it!
Ray AD is constructed to be a bisector of angle CAB.
Since ABC is equilateral, m∠CAB = 60 degrees. Therefore, m∠CAD = 30 degrees, which is half of ∠CAB.
If m LZ = 56°, determine and state the degree measure of angle P.
K is the center of the circle, so LE is a diameter splitting the circle into two semicircles.
This means that arc EZL has a measure of 180 degrees, of which arc LZ is 56 degrees.
Therefore, arc EZ is 180 - 56 = 124 degrees.
1/2 (124 - 56) = 1/2 (68) = 34 degrees.
Angle P + 56 + 90 = 180, so Angle P = 34 degrees.
You want to find the volume of the cylinder, but to a height of 2 1/2 feet instead of 3 feet. The radius is half of the diameter, which is (1/2)(8 1/4) = 4 1/8
Use the pi key on your calculator to get extra decimals for pi. Do NOT use 3.14 because it will not be accurate enough.
V = pi * r2 * h
V = 3.141592... * (4.125)2 * 2.5 = 133.64 = 134 cubic feet.
Monday, August 26, 2019
August 2019 Algebra 1 Regents Parts 3 & 4
The following are some of the multiple questions from the August 2019 New York State Common Core Algebra I Regents exam.
Each correct answer is worth up to 4 credits. Partial credit can be given. Work must be shown or explained.
33.
On the set of axes below, graph the following system of inequalities:
Answer:
August 2019 Algebra I, Part III
y - 5 < 3x
Determine if the point (1,8) is in the solution set. Explain your answer.
Rewrite the equations in slope-intercept form
y - 5 < 3x + 5
Note that the first is greater than or equal to, so it will be a solid line that is shaded above.
The second is less than, so it will be a broken or dashed line that is shaded below.
If you are not sure which side to shade, substitute the point (0, 0) in the inequality. If you get a true statement as a result, then shade that side. If the statement is false, shade the other side.
IMPORTANT: this test doesn't work if the line goes through (0, 0). In that case, pick any other point not on the line. It will work for any point, but (0, 0) is the easiest one to use!
The first line (solid) has a slope of -2 and a y-intercept of 8.
The second line (broken) has a slope of 3 and a y-intercept of 5.
The point (1, 8) is NOT part of the Solution Set because it is on the broken line, which is a boundary, but not a part of the solution.
Important: if you have a graphing error, then your response to this part of the question should match your graph to get the point.
34.
On the day Alexander was born, his father invested $5000 in an account with a 1.2% annual
growth rate. Write a function, A(t), that represents the value of this investment t years after
Alexander’s birth.
Determine, to the nearest dollar, how much more the investment will be worth when Alexander
turns 32 than when he turns 17.
Answer:
For the first part, you have to write a formula.
For the second part, you have to use the formula twice for when he is 32 and 17, and subtract the difference.
A(t) = 5000 (1.012)t
A(32) - A(17) = 5000 (1.012)32 - 5000 (1.012)17 = 1199.91758695
$1200.
You can put that entire equation in your calculator. You do not need to evaluate the parts separately.
35.
Stephen collected data from a travel website. The data included a hotel’s distance from Times
Square in Manhattan and the cost of a room for one weekend night in August. A table containing
these data appears below.
Write the linear regression equation for this data set. Round all values to the nearest hundredth.
State the correlation coefficient for this data set, to the nearest hundredth.
Distance From
Times Square
(city blocks) (x)0 0 1 1 3 4 7 11 14 19
Cost of
a Room
(dollars) (y) 293 263 244 224 185 170 219 153 136 111
Answer:
Enter the data into two lists, L1 and L2.
Make sure DiagnosticOn has been set. (If you don't know, just do select it from the Menu.)
Press STAT to get to the statistics menu and arrow over to CALC. Choose option 4, LinReg(ax+b).
You will get a = -7.76, b = 246.34 and r = -.88, rounded to the nearest hundredth.
The linear regression is y = -7.76x + 246.34
The correlation coefficient is r = -0.88
In the context of this problem, the correlation coefficient suggest a strong negative correlation between the number of blocks from Times Square and the cost of a room. As you get farther away from Times Square, the cost of the room decreases.
(It's not enough to just say that it's a strong negative correlation.)
Coincidentally, this question is very similar to Question 35 of the June 2019 Regents. I barely had to do any editing to my solution (which, yes, I cut and pasted from the old post because all the formatting is in place).
36.
A snowstorm started at midnight. For the first 4 hours, it snowed at an average rate of one-half
inch per hour.
Determine the average rate of snowfall over the length of the storm. State the rate, to the nearest
hundredth of an inch per hour.
The snow then started to fall at an average rate of one inch per hour for the next 6 hours.
Then it stopped snowing for 3 hours.
Then it started snowing again at an average rate of one-half inch per hour for the next 4 hours until
the storm was over.
On the set of axes below, graph the amount of snow accumulated over the time interval of the
storm.
Answer:
Notice that the scale for the x-axis is 1 box = 1 hour, but the scale for the y-axis is 1 box = 1/2 inch of snow. Don't let that confuse you.
You can plot points and draw line segments just by counting the boxes. The thing to remember is if no snow falls, the accumulation doesn't change. Slope is 0 (flat line) for that time period.
To find the average, what would the slope be between the origin (0,0) and the highest point on the graph at the end of the storm, which should be (17, 10)?
(10 - 0) / (17 - 0) = 10 / 17 = 0.5882... = 0.59 inches per hour (to the nearest hundredth).
Again, if you made a graphing error, use whatever point you finished at.
For example, if you missed a point when it stopped for 3 hours (suppose you graphed 2), and you ended up at (16, 10), you would have done 10/16 = 0.625, which is 0.63. You get credit if you are consistent.
August 2018 Algebra I, Part IV
A correct answer is worth up to 6 credits. Partial credit can be given. Work must be shown or explained.
37.
Allysa spent $35 to purchase 12 chickens. She bought two different types of chickens. Americana
chickens cost $3.75 each and Delaware chickens cost $2.50 each.
Write a system of equations that can be used to determine the number of Americana chickens, A,
and the number of Delaware chickens, D, she purchased.
Determine algebraically how many of each type of chicken Allysa purchased.
Each Americana chicken lays 2 eggs per day and each Delaware chicken lays 1 egg per day.
Allysa only sells eggs by the full dozen for $2.50. Determine how much money she expects to take
in at the end of the first week with her 12 chickens.
Answer:
A + D = 12
3.75A + 2.50D = 35
You can solve by Sustitution (Substitute 12 - A for D) or Elimination (multiply the first equation by -2.50).
A + D = 12
3.75A + 2.50D = 35
3.75A + 2.50(12 - A) = 35
3.75A + 30 - 2.50 = 35
1.25A + 30 = 35
1.25A = 5
A = 4
4 + D = 12
D = 8
4 American and 8 Delaware
Or
A + D = 12
3.75A + 2.50D = 35
-2.50A - 2.50D = 30
1.25A + 30 = 35
1.25A = 5
A = 4
4 + D = 12
D = 8
4 American and 8 Delaware
The number of eggs laid per day are 4(2) + 8(1) = 16.
After one week, she will have 16 * 7 = 112 eggs
This makes 112 / 12 = 9.333.... dozen.
She can sell 9 dozen for $2.50 each, which is 9 * 2.50 = $22.50.
I would hate to have to follow through the work, checking for consistency, if the number of chickens was incorrect!
End of Exam
How did you do?
Questions, comments and corrections welcome.
Sunday, August 25, 2019
August 2019 Algebra 1 Regents, Part 2
The following are some of the multiple questions from the August 2019 New York State Common Core Algebra I Regents exam.
Each correct answer is worth up to 2 credits. Partial credit can be given. Work must be shown or explained.
25.
If g(x) = -4x2 - 3x + 2, determine g(2).
Answer:
One point off for one computational error, such as (-2)2 = -4, or similar.
26.
A student is in the process of solving an equation. The original equation and the first step are
shown below.
Answer:
27.
On the set of axes below, graph the line whose equation is 2y = -3x - 2.
Answer:
You can plainly see that the point (2, k) is (2, -4), so k = -4.
If you graphed this incorrectly, but your answer for k matches your graph, you will get credit for that portion.
28.
The formula a = (vf - vi) / t is used to calculate acceleration as the change in velocity over the period of time.
Answer:
a = (vf - vi) / t
29.
Solve 3/5 x + 1/3 < 4/5 x - 1/3 for x.
Answer:
3/5 x + 1/3 < 4/5 x - 1/3
30.
Is the product of two irrational numbers always irrational? Justify your answer.
Answer:
31.
Solve 6x2 - 42 = 0 for the exact values of x.
Answer:
6x2 - 42 = 0
If you go any further than that, you will lose a point, so STOP.
If you used the Quadratic Formula, you would have gotten something like + SQRT(1008) / 12. This is OKAY because that is an Exact value, which can be simplified into 12 * SQRT(7) / 12, but you wouldn't be required to do this. However, if you did attempt to simplify it, you would have to get it right.
32.
Graph the function:
Answer:
End of Part II
How did you do?
Questions, comments and corrections welcome.
August 2019 Algebra I, Part II
Substituion:
g(2) = -4(-2)2 - 3(-2) + 2
= -4(4) - (-6) + 2
= -16 + 6 + 2 = -8
Original: 3a + 6 = 2 - 5a + 7
Step one: 3a + 6 = 2 + 7 - 5a
The student used the Commutative Property to switch the order of the last to terms, which allows the student to Combine Like Terms in the next step.
This linear equation contains the point (2,k). State the value of k
Divide the equation by 2 to get it in slope-intercept form. You will see that the y-intercept is -1 and the slope is -3/2. You could use the graphing calculator at this point, or you can plot the point (0, 11) and then make you next point down 3 spaces and 2 to the right, and then repeat. When you get to the edge of the graph, go back the other way: 3 spaces up and 2 to the left, and repeat. And the arrows.
If you used the equation 2y = -3(2) - 2 to find the y-coordinate, you will probably get the credit.
Solve the formula for the final velocity, vf, in terms of initial velocity, vi, acceleration, a, and time, t.
This is a real-world problem: calculating a final velocity when given an initial velocity, acceleration and a period of time.
Isolate the variable by moving everything else to the other side of the equation.
at =vf - vi
at + vi = vf
You can eliminate the fractions, if you want, by multiplying the entire equation by 15. Or you can simplify it a little first.
2/3 < 1/5 x
(15)(2/3) < (1/5 x)(15)
10 < 3x
10/3 < x
No, sometimes the answer is rational. SQRT(2) * SQRT(2) = 2, which is rational.
Exact values of x means that you do NOT estimate irrational numbers. Do not round anything.
6x2 = 42
x2 = 7
x = +SQRT(7)
x2 - 4x - 5, 0 < x < 5
ONLY Graph up to x = 5 and no farther. No arrow. The left side, however, does get an arrow. The left portion ends with an OPEN circle. The parabola starts with a CLOSED circle. You could end it with a closed circle as well, if you want, or just a point.
Friday, August 23, 2019
August 2019 Algebra 1 Regents Part 1
The following are some of the multiple questions from the recent August 2019 New York State Common Core Algebra I Regents exam.
Omitted images will be added soon.
Each correct answer is worth up to 2 credits. No partial credit. Work need not be shown.
1. Bryan’s hockey team is purchasing jerseys. The company charges $250
for a onetime set-up fee and $23 for each printed jersey. Which
expression represents the total cost of x number of jerseys for the
team?
Answer: (3) 23x + 250
2. Which table represents a function?
Answer: (4)
3. Which expression is equivalent to 2(x2 - 1) + 3x(x - 4)?
Answer: (4) 5x2 - 12x - 2
August 2019 Algebra I, Part I
The start-up fee is paid once, so it doesn't get a variable. It's the cost even if 0 jerseys are made, so it's the y-intercept of a graph. The $23 is a repeated cost per jersey, so it gets a variable.
Choices (1), (2) and (3) each repeat an x value with a different y value. That is not allowed in a function.
2(x2 - 1) + 3x(x - 4)
2x2 - 2 + 3x2 - 12x
5x2 - 12x - 2
4. The value of x that satisfies the equation 4/3 = (x + 10) / 15 is
Answer: (3) 10
The quick way: 3 goes into 15 five times, and 4 * 5 = 20 = x + 10. Then x = 10.
or
4/3 = (x + 10) / 15
(3)(x + 10) = (4)(15)
3x + 30 = 60
3x = 30
x = 10
5. Josh graphed the function f(x) = -3(x - 1)2 + 2. He then graphed the function g(x) = -3(x - 1)2 - 5 on the same coordinate plane. The vertex of g(x) is
Answer: (1) 7 units below the vertex of f(x)
The vertex of f(x) is (1, 2). The vertex of g(x) is (1, -5).
Therefore the vertex of g(x) is 7 units lower than f(x).
6. A survey was given to 12th-grade students of West High School to
determine the location for the senior class trip. The results are shown
in the table below.
Niagara Falls Darien Lake New York City Boys 56 74 103 Girls 71 92 88
To the nearest percent, what percent of the boys chose Niagara Falls?
Answer: (2) 24
Sum the number of boys by adding the three responses: 56 + 74 + 103 = 233.
Divide the number for Niagara Falls by 233, and make it a percent: 56 / 233 * 100% = 24%
Note that you don't need to use any of the data for the girls.
7. Which type of function is shown in the graph below?
Answer: (2) exponential
Basically, a definition question. Linear functions and absolute value functions are straight lines, not curves.
A square root bends the other way, with smaller increases in y and x gets larger.
8. The expression 16x2 - 81 is equivalent to
Answer: (3) (4x - 9)(4x + 9)
The Difference of Squares Rule: when you have the difference between two terms which are each perfect squares, take the square root of each term and write the conjugates. (That is, write the two terms twice, once with a plus between them, and once with a minus.)
9. The owner of a landscaping business wants to know how much time, on average, his workers spend mowing one lawn. Which is the most appropriate rate with which to calculate an answer to his question?
Answer: (4) hours per lawn
Time per lawn: time can be measured in hours and lawns in the unit measurement.
Lawns per day doesn't give the amount of time for one lawn.
10. A ball is thrown into the air from the top of a building. The height, h(t), of the ball above the ground t seconds after it is thrown can be modeled by h(t) = -16t2 + 64t + 80. How many seconds after being thrown will the ball hit the ground?
Answer: (1) 5
Put the equation in your graphing calculator, and check the table. It has zeroes at -1 and 5. Reject -1 because negative time makes no sense.
The long way:
-16t2 + 64t + 80 = 0 -- Divide by -16 to get smaller numbers.
t2 - 4t - 5 = 0
(t - 5)(t + 1) = 0
t = 5 or t = -1, reject -1
11. Which equation is equivalent to y = x2 + 24x - 18?
Answer: (1) y = (x + 12)2 - 162
You don't have to complete the square because choices are given.
Actually, you wouldn't have to complete the square even without the choices.
Put the graph into the calculator and look at the table. Where is the vertex? It is at (-12, -162).
You can also see that (-12, 126), (12, 162) and (12, 126) are not even points on the parabola.
Next method: the vertex is on the Axis of Symmetry, so x = -b / (2a) = -24/(2*1) = -12.
Eliminate choices (3) and (4).
Then y = (-12)2 + 24(-12) - 18 = -162.
12. When (x)(x - 5)(2x + 3) is expressed as a polynomial in standard form, which statement about the resulting polynomial is true?
Answer: (2) The leading coefficient is 2.
(x)(x)(2x) = 2x3, which is be the leading term, with the highest exponent. This also means that the degree is 3, and not 2.
The constant will be (-5)(3) = -15, not 2. And there will be more than these 2 terms.
13. The population of a city can be modeled by P(t) = 3810(1.0005)7t , where P(t) is the population after t years. Which function is approximately equivalent to P(t)?
Answer: (2) P(t) = 3810(1.0035)t
(1.0005)7t = (1.00057)t.
1.0005 to the 7th power is approximately 1.0035.
The rest of the equation remains unchanged.
14. The functions f(x) and g(x) are graphed on the set of axes below.
For which value of x if f(x) =/= g(x)?
Answer: (3) 3
The graphs intersect at x = -2, x = -1 and x = 2. At x = 3, g(3) = 0, but f(3) is off the top of the graph.
15. What is the range of the box plot shown below?
Answer: (1) 7
The range is 8 - 1 = 7.
The difference 6 - 4 (which is 2) would be the Interquartile Range, which is different.
16. Which expression is not equivalent to 2x2 + 10x + 12?
Answer: (3) (2x + 3)(x + 4)
Not to be a broken record, but you could put each of these into the graphing calculator and see if they give you the same graph. However, in this case, that would actually take more time.
Just looking at the choices, you should immediately see that (2) and (4) are equivalent to each other because (2x + 6) is the same as 2(x + 3). So neither of those in the answer.
Likewise, there is no way that both (1) and (3) could be equivalent: switching the 3 and 4 will result in a different middle term. So check those:
(2x + 4)(x + 3) = 2x2 + 6x + 4x + 12 =
2x2 + 10x + 12. Check!
(2x + 3)(x + 4) = 2x2 + 8x + 3x + 12 =
2x2 + 11x + 12. Wrong!
17. The quadratic functions r(x) and q(x) are given below
q(x)=x2 + 2x - 8 The function with the smallest minimum value is
x r(x) -4 -12 -3 -15 -2 -16 -1 -15 0 -12 1 7
Answer: (3) r(x), and the value is -16
The minimum for r(x) is -16.
Graph q(x) and find the vertex. Or use the Axis of Symmetry, x = -2/(2(1)) = -1.
Evaluate q(-1) = (-1)2 + 2(-1) - 8 = 1 - 2 - 8 = -9.
The function with the smallest minimum value is r(x), and that value is -16 (which occurs when x = -2, but that is NOT what is being asked).
18. A child is playing outside. The graph below shows the child's distance, d(t), in yards from home over a period of time, t, in seconds.
Which interval represents the child constantly moving closer to home?
Answer: (1) 0 < t < 2
If the child is moving toward home at a constant rate, the distance is decreasing steadily.
That happens between 0 and 2 seconds. at you're accidentally get the "right" answer to appear by mistake.
19. If a1 = 6 and an = 3 + 2(an - 1)2, the a2 equals
Answer: (1) 75
a2 = 3 + 2(a2 - 1)2 = 3 + 2(a1)2
= 3 + 2(6)2 = 3 + 2(36) = 3 + 72 = 75
20. The length of a rectangular patio is 7 feet more than its width, w. The area of a patio, A(w), can be represented by the function
Answer: (2) A(w) = w2 + 7w
Length = w + 7. Area = length * width = (w + 7)(w) = w2 + 7w.
21. A dolphin jumps out of the water and then back into the water. His jump could be graphed on a set of axes where x represents time and y represents distance above or below sea level. The domain for this graph is best represented using a set of
Answer: (4) positive real numbers
Forget about the above and below the water. They asked for the domain, which is the x value, which in this case represents time. Negative time makes no sense.
I don't like this problem because it leaves out 0, which is not positive, but should be part of the domain. However, the answer key tells me that it is not part of the domain.
22. Which system of linear equations has the same solution as the one
shown below?
x - 4y = 10
x + y = 5
Answer: (1) 5x = 10, x + y = 5
Change x + y = 5 into y = 5 - x
That makes the first equation x - 4(5 - x) = -10
and then x - 20 + 4x = -10
So 5x - 20 = -10
And 5x = 10, which is choice (1).
23. Which interval represents the range of the function h(x) = 2x2 - 2x - 4?
Answer: (4) [4.5, ∞)
It's a parabola that opens upwards, so the upper boundary is infinity. The lower boundary is the minimum point at the vertex. Just looking at the choices, the answer should be obvious once you realize what the incorrect answer is.
You can graph this and look for the vertex.
Or use the Axis of Symmetry: x = -b/(2a) = -(-2)/(2(2)) = 0.5, which is NOT the range.
h(.5) = 2(.5)2 - 2(.5) - 4 = -4.5, which is part of the range, so use [ not (.
24. What is a common ratio of the geometric sequence whose first term is 5 and third term is 245?
Answer: (1) 7
The second term must be n/5 = 245/n, so n2 = (5)(245)
n = SQRT(1225) = 35
35 / 5 = 7, and 245 / 35 = 7
The Common ratio is 7.
End of Part I
How did you do?
Questions, comments and corrections welcome.
Wednesday, August 21, 2019
Dollars to Doughnuts
(C)Copyright 2019, C. Burke.
It's an old saying, so I went with an old spelling.
When I was a kid, $6 vs 6 donuts would have been about 3:1 odds or so. Now, it could be 1:2 or 1:3 or worse, depending on where you shop and the kind of donuts.
Come back often for more funny math and geeky comics.
Monday, August 19, 2019
Caught in a Trap
(C)Copyright 2019, C. Burke.
Y can't U C?
Previous mentions and appearances by LVI were Agent 56 Lives! and 56 is 75.
No need for suspicious minds.
Come back often for more funny math and geeky comics.
Saturday, August 17, 2019
Dance With Fire
(C)Copyright 2019, C. Burke.
Exploit the weakness. Get them talking.
The things you do for love, right? Anyway, the idea for this was might go as far back as The Temple of Duran, and it was definitely meant to be a follow-up to Off On a Tangent, which I didn't get around to doing.
Come back often for more funny math and geeky comics.
Tuesday, August 13, 2019
Tucker
(C)Copyright 2019, C. Burke.
You'll miss your honey. You'll feel so lonely.
Come back often for more funny math and geeky comics.
Thursday, August 08, 2019
A Quick High
(C)Copyright 2019, C. Burke.
Posted on Facebook a few years back. Along with a similar response.
EDIT: Changed the wording to what I'd originally intended, replacing "quickly" with "too fast". I think it reads better, even if the grammar is poorer.
Come back often for more funny math and geeky comics.
Wednesday, August 07, 2019
(x, why?) Mini: Vote!
(C)Copyright 2019, C. Burke.
There are four sides to his platform.
Come back often for more funny math and geeky comics.
Thursday, August 01, 2019
Who Can Dance If They Want To
(C)Copyright 2019, C. Burke.
I was going to use Truth tables and conditionals, but the "leave your friends behind" statement was a dead giveaway.
Come back often for more funny math and geeky comics.