Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2011

Part I: Each correct answer will receive 2 credits.


6. What is the value of x in the equation log₅ x = 4?

1) 1.16
2) 20
3) 625
4) 1,024

Answer: 3) 625

If log base 5 of x is 4 then 5 to the power of 4 is x.

5⁴ = 625, which is choice (3).

Choice (1) is the approximate answer to 4^x = 5.

Choice (2) is just 4 times 5.

Choice (4) is 4⁵, if you reversed the expression that you wanted.

7. The expression ⁴√(16x²y⁷ is equivalent to

1) 2x^1/2y^7/4
2) 2x⁸y²⁸
3) 4x^1/2y^7/4
4) 4x⁸y²⁸

Answer: 1) 2x^1/2y^7/4

The fourth root of 16 is 2 because 2⁴ = 16. Eliminate Choices (3) and (4).

The 4th root is the same as taking the 1/4 power, so divide the exponents under the radical by 4, and you get 1/2 and 7/4. This is Choice (1).

DON'T multiply them.

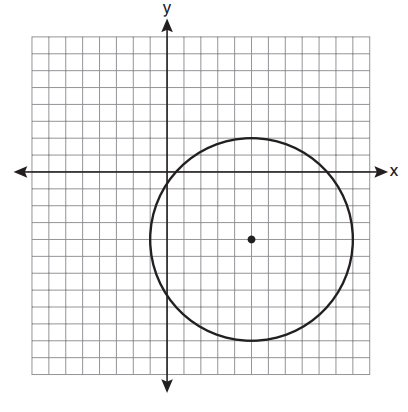
8. Which equation is represented by the graph below?

1) y = 5^x
2) y = 0.5^x
3) y = 5^-x
4) y = 0.5^-x

Answer: 2) y = 0.5^x

Choices (1) and (4) would both be increasing functions, not decreasing, so eliminate them.

(0.5)^x = (1/2)^x and (5)^-x = (1/5)^x

If you look at x = -1, y = 2, and (1/2)^(-1) = 2. The answer is Choice (1).

9. What is the fifteenth term of the geometric sequence −√(5), √(10), −2√(5), . . . ?

1) −128√(5)
2) 128√(10)
3) −16384√(5)
4) 16384√(10)

Answer: 1) −128√(5)

The common ratio in the sequence is √(10)/-√(5) = -√(2). First, you can see that the 15th term will be negative, so eliminate Choices (2) and (4).

The 50th term would be -√(5)*(-√(2))¹⁴, which is -√(5)*(2)⁷, which is -128√(5).

10. In △ABC, a = 15, b = 14, and c = 13, as shown in the diagram below. What is the m∠C, to the nearest degree?

1) 53
2) 59
3) 67
4) 127

Answer: 1) 53

The angle across from the smallest side is the smallest angle. The triangle is 13-14-15, which is almost equilateral, so the angles are close to 60, but angle C must be smaller than 60. Eliminate Choices (3) and (4).

The question is does the difference in sides reduce the angle all the way down to 53 degrees? My hunch before working out this problem is that 53 is way more likely than 59.

Since we don't know any of the angles, we aren't going to use the Law of Sines. Instead, we'll use the Law of Cosines

cos(C) = (a² + b² − c²) / (2ab)

cos(C) = (15² + 14² − 13²) / (2(14)(15))

cos(C) = 3/5

C = cos^-1 3/5 = 53.1 degrees

Looks like my hunch was good, but I'm glad I worked it out anyway.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

EDIT: I don't know what exam I started below, but it doesn't appear to be August 2011. It will be corrected soon.

More Regents problems.

Geometry Regents, June 2011

Part I: Each correct answer will receive 2 credits.


6. In the diagram below, line p intersects line m and line n.

If m∠1 = 7x and m∠2 = 5x + 30, lines m and n are parallel when x equals

1) 12.5
2) 15
3) 87.5
4) 105

Answer: 2) 15

It should be pretty obvious from the Choices that (3) and (4) are the angle sizes and that either (1) or (2) is the value of x we want.

Angles 1 and 2 are congruent because they are alternate interior angles. They are NOT supplementary. So you want to write an equation that says that they are equal to each other, not one that sums them to 180.

7x = 5x + 30

2x = 30

x = 15

7. In the diagram of △KLM below, m∠L = 70, m∠M = 50, and MK is extended through N.

What is the measure of ∠LKN ?

1) 60°
2) 120°
3) 180°
4) 300°

Answer: 2) 120°

Choices (3) and (4) are just bizarre. Cross them out immediately.

The Remote Angle Theorem tells you that ∠LKN is the sum of the two given angles, 70 + 50 = 120.

If you didn't remember that theorem, you could do it the long way: angle LKM must be 60 degrees, because the three angles of a triangle must add up to 180. Then LKN must be supplementary to the 60 degree angle, and 180 - 60 = 120. Same result. Choice (2).

8. If two distinct planes, A and B, are perpendicular to line c, then which statement is true?

1) Planes A and B are parallel to each other.
2) Planes A and B are perpendicular to each other.
3) The intersection of planes A and B is a line parallel to line c.
4) The intersection of planes A and B is a line perpendicular to line c.

Answer: 1) Planes A and B are parallel to each other.

Consider a fire pole from floor 2 to floor 1 in a firehouse. Or consider an elevator, but that isn't as much fun as a fire pole.

You can also think of a a line running across a floor from one wall to a parallel wall opposite it. Or a shoebox. Or anything similar. The planes will not intersect each other.

9. What is the length of the line segment whose endpoints are A(−1,9) and B(7,4)?

1) √(61)
2) √(89)
3) √(205)
4) √(233)

Answer: 2) √(89)

Use Pythagorean Theorem or the Distance Formula. (You can make a sketch on the scratch graph paper and count the boxes. Whichever.)

From -1 to 7 is a distance of 8 units. From 9 to 4 is a distance of 5 units. (This is length, so we don't want a negative sign).

8^2 = 64 and 5^2 = 25; and 64 + 25 = 89.

So the length is √(89).

10. What is an equation of circle O shown in the graph below?

1) (x + 1)² + (y − 3)² = 25
2) (x - 1)² + (y + 3)² = 25
3) (x - 5)² + (y + 6)² = 25
4) (x + 5)² + (y − 6)² = 25

Answer: 1) (x + 1)² + (y − 3)² = 25

The equation for a circle is given by the formula (x - h)² + (y - k)² = r², where (h,k) is the center of the circle and r is the radius.

The center of the circle is (-1,3), so eliminate Choices (3) and (4). Since there are minus signs in the formula, the signs get flipped. The -1 becomes (x + 1) and the 3 becomes (x - 3). This is Choice (1).

Since r² is 25 is all four choices, you don't have to calculate the radius from the two points.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2011

Part I: Each correct answer will receive 2 credits.


1. A doctor wants to test the effectiveness of a new drug on her patients. She separates her sample of patients into two groups and administers the drug to only one of these groups. She then compares the results. Which type of study best describes this situation?

1) census
2) survey
3) observation
4) controlled experiment

Answer: 4) controlled experiment

This is a definition question. The question described how to conduct a controlled experiment.

2. If f(x) = x / (x² - 16), what is the value of f(-10)?

1) -5/2
2) -5/42
3) 5/58
4) 5/18

Answer: 2) -5/42

Substitute and evaluate. Simplify your fraction so that it matches one of the choices.

f(-10) = -10 / (100 - 16). The answer is negative, so eliminate Choices (3) and (4).

f(-10) = -10/84 = -5/42, which is Choice (2).

3. An auditorium has 21 rows of seats. The first row has 18 seats, and each succeeding row has two more seats than the previous row. How many seats are in the auditorium?

1) 540
2) 567
3) 760
4) 798

Answer: 4) 798

There is an even number of seats, so eliminate Choice (2).

There are 18 seats in the first row, 20 in the second, 22 in the third, and 18 + 2(20) = 58 seats in the last row.

You can add those numbers or take a shortcut. 18 + 58 = 76, 76 * 10 = 760, 760 + 1/2(76) = 798, which is Choice (4).

4. Expressed as a function of a positive acute angle, cos (−305°) is equal to

1) −cos 55°
2) cos 55°
3) −sin 55°
4) sin 55°

Answer: 2) cos 55°

There are 360 degrees in a complete circle. Moving -305 degrees is the same as moving positive 55 degrees. So the answer is cos 55. There's no need to switch signs or functions.

5. The value of x in the equation 4^{2x + 5} = 8^3x is

1) 1
2) 2
3) 5
4) -10

Answer: 2) 2

This is a siple enough problem that you could just substitute and evaluate. However, it is a good problem wo solve because this is a decent open-ended question if you had no choices:

4 is 2² and 8 is 2³, so rewrite the equation using a base of 2. Then set the exponent expressions equal to each other.

2^{2(2x + 5)} = 2^(3)(3x)

4x + 10 = 9x

10 = 5x

x = 2

Check: 4^{2(2) + 5} = 4⁹ = 262144; 8³⁽²⁾ = 8⁶ = 262144. Check!

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, June 2011

Part I: Each correct answer will receive 2 credits.


6. What is 3√(250) expressed in simplest radical form?

1) 5√(10)
2) 8√(10)
3) 15√(10)
4) 75√(10)

Answer: 3) 15√(10)

It is obvious that 25 is a factor of 250. Is it the largest perfect square that is a factor of 250? Yes, 250 = 25 * 10, and 10 does not have any perfect squares as factors.

An alternative way to look at this is that 250 = 2 * 5 * 5 * 5, which means that you can remove one pair of factors from under the square root symbol.

So 3 √(250) = 3 * √(25) * √(10) = 3 * 5 * √(10) = 15√(10).

7. A survey is being conducted to determine which school board candidate would best serve the Yonkers community. Which group, when randomly surveyed, would likely produce the most bias?

1) 15 employees of the Yonkers school district
2) 25 people driving past Yonkers High School
3) 75 people who enter a Yonkers grocery store
4) 100 people who visit the local Yonkers shopping mall

Answer: 1) 15 employees of the Yonkers school district

Looking for the most bias, Choice (1) has a combination of the fewest people surveyed (and 15 is a very small sample size) and the population is the most limited, oversampling for one specific demographic (school district employees).

8. An 8-foot rope is tied from the top of a pole to a stake in the ground, as shown in the diagram below.

If the rope forms a 57° angle with the ground, what is the height of the pole, to the nearest tenth of a foot?

1) 4.4
2) 6.7
3) 9.5
4) 12.3

Answer: 2) 6.7

You have a right triangle. You know the length of the hypotenuse. You are looking for the side opposite of the known angle. That means that you need to use the sine function.

sin 57 = x / 8

x = 8 * sin 57 = 6.7 ..., which is choice (2).

Choice (1) is the result if you used cosine by mistake.

Choices (3) and (4) both larger than the hypotenuse and can be immediately eliminated. These answers from writing hypotenuse over opposite (or over the adjacent) and solving the proportion incorrectly.

9. How many different ways can five books be arranged on a shelf ?

1) 5
2) 15
3) 25
4) 120

Answer: 4) 120

There are 5! permutations, which is 5! = 5 * 4 * 3 * 2 * 1 = 120.

10. What is the slope of the line passing through the points (-2,4) and (3,6)?

1) -5/2
2) -2/5
3) 2/5
4) 5/2

Answer: 3) 2/5

The x values are increasing and the y values are increasing, so the slope is positive. Eliminate Choices (1) and (2).

You want the change in the rise over the run, which is the change in y over the change in x.

6 - 4 = 2 and 3 - (-2) = 5, so the slope is 2/5, which is Choice (3).

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

(x, why?) Mini: After Christmas Trees

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

In a world of shapes, forming a Christmas tree would be like putting on a play. And the play's over.

In the real world, trees usually stay up a bit longer.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2011

Part I: Each correct answer will receive 2 credits.


1. Line segment AB is shown in the diagram below.

Which two sets of construction marks, labeled I, II, III, and IV, are part of the construction of the perpendicular bisector of line segment AB?

1) I and II
2) I and III
3) II and III
4) II and IV

Answer: 2) I and III

To construct a perpendicular bisector, from each endpoint, make an arc above and below the line so that they intersect. That would be points I and III, which is Choice (2).

2. If △JKL ≅ △MNO, which statement is always true?

1) ∠KLJ ≅ ∠NMO
2) ∠KJL ≅ ∠NMO'
3) JL ≅ MO
4) JK ≅ ON

Answer: 3) JL ≅ MO

Contrary to what many overworked math teachers may write on the blackboard (or whiteboard, or whatever), when stating that two triangles are congruent, the vertices are supposed to correspond in the order that they are written.

This means that writing △DOG ≅ △CAT is not the same as writing △DOG ≅ △ACT

So we can assume that J corresponds to M, etc.

Choice (1) is incorrect because K doesn't correspond to M. (The central letter of the angle is the one that's most important.)

Choice (2) is inccorrect because J doesn't correspond to O.

Choice (3) is correct because J goes to M and L goes to O.

Choice (4) is incorrect because J goes to M and M isn't either endpoint on the right side.

3. In the diagram below, △A′B′C′ is a transformation of △ABC, and △A″B″C″ is a transformation of △A′B′C′.
The composite transformation of △ABC to △A″B″C″ is an example of a

1) reflection followed by a rotation
2) reflection followed by a translation
3) translation followed by a rotation
4) translation followed by a reflection

Answer: 4) translation followed by a reflection

The triangle moved down and to the right before being flipped over the y-axis. That is a translation followed by a reflection.

4. In the diagram below of △ACE, medians AD, EB, and CF intersect at G. The length of FG is 12 cm.
What is the length, in centimeters, of GC?

1) 24
2) 12
3) 6
4) 4

Answer: 1) 24

CG is the centroid, the concurrence point for three medians in a triangle. CG is 2/3 the length of CF and FG is 1/3 of the length of CF.

You are given that FG is 12, so GC is twice as large (2/3 vs 1/3), or 24.

Choice (2) assumes the segments are congruent, when they are not.

Choice (3) assumes you thought CG was 12 and they asked for GF.

Choice (4) assumes you thought CF was 12 and they asked for GF.

5. In the diagram below of circle O, chord AB is parallel to chord CD.

Which statement must be true?

1) arc AC ≅ arc BD
2) arc AB ≅ arc CD
3) segment AC ≅ segment CD
4) arc ABD ≅ arc CDB

Answer: 1) arc AC ≅ arc BD

If two chords are parallel, then the arcs that they intercept will be congruent. That is choice (1).

Choices (2), (3) and (4) will only happen if the two lines are parallel and equidistant from the center of the circle. In that case, all four statements would be true.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, June 2011

Part I: Each correct answer will receive 2 credits.


1. The expression x² - 36y² is equivalent to

1) (x - 6y)(x - 6y)
2) (x - 18y)(x - 18y)
3) (x + 6y)(x - 6y)
4) (x + 18y)(x - 18y)

Answer: 3) (x + 6y)(x - 6y)

When we have the Difference of Two Perfect Squares, it can be factored into two binomials which are conjugates. Take the sqaure roots of each of the term.

That is choice (3).

Choice (1) would be x² - 12xy + 36y².

Choices (2) and (4) have 18, which is half of 36, instead of 6, which is the square root.

2.The legs of an isosceles right triangle each measure 10 inches. What is the length of the hypotenuse of this triangle, to the nearest tenth of an inch?

1) 6.3
2) 7.1
3) 14.1
4) 17.1

Answer: 3) 14.1

You can use Pythagorean Theorem to find the answer if you didn't know that the hypotenuse in an right isosceles triangle is the legnth of one leg times SQRT(2).

10 * 1.414... = 14.14...

3. The expression (12w⁹y³) / (-3w³y³) is equivalent to

1) -4w⁶
2) -4w³y
3) 9w⁶
4) 9w³y

Answer: 1) -4w⁶

Divide the coeffients and subtract the exponents of the like variables.

12/(-3) = -4, so eliminate Choices (3) and (4).

w⁹ / w³ = w9 - 3 = w6. This is Choice (1).

4. The spinner shown in the diagram below is divided into six equal sections.

1) an odd number
2) a prime number
3) a perfect square
4) a number divisible by 2

Answer: 3) a perfect square

Which category has the fewest members n the group of integers from 1 to 6?

There are 3 odd numbers.

There are 3 prime numbers.

There are 2 perfect sqaures.

There are 3 numbers divisible by 2 (even numbers).

So perfect squares are the least likely.

5. What are the factors of the expression x² + x - 20?

1) (x + 5) and (x + 4)
2) (x + 5) and (x - 4)
3) (x - 5) and (x + 4)
4) (x - 5) and (x - 4)

Answer: 2) (x + 5) and (x - 4)

The -20 tells us that the factors have different signs. The + x tells us that the larger factor is has a plus sign. That is Choice (2).

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Merry Christmas 2021!

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

Merry Christmas!

This wasn't the Christmas week or Christmas season I envisioned -- I can't even say "planned" because I hadn't really come up with a plan. There might've been two ideas for comics that didn't happen, but not much solid. I usually enjoy doing this comics and coming up with something new by Christmas Eve. Unfortunately, that didn't pan out.

Today's comic isn't much, but it's more than it would've been had I posted it yesterday morning.

For anyone who celebrated, I hope your Christmas Eve was a happy, healthy and safe one, full of fishes or not. (Yeah, a Seven fishes jokes would've been nice.)

Merry Christmas! Updates next week, and into the New Year! Let's hope it's a better one than the last one.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Christmas Past

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

And there was born a child, who would grow to be a human aerial. As did his brother.

Such was life as the youngest sibling in an old aparment with an old TV set and no antenna on the roof.

The picture was usually snowy, and you were lucky if you could a hold on a good signal.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, August 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, August 2011

Part III: Each correct answer will receive 4 credits. Partial credit is available.


35. In the diagram below of △GJK, H is a point on GJ, HJ ≅ JK , m∠G = 28, and m∠GJK = 70.
Determine whether △GHK is an isosceles triangle and justify your answer

Answer:

Mark HJ and JK so you remember which two lines are congruent. Don't mark HK by mistake.

Angle J is the vertex angle, and is 70 degrees. So the other two angles in HJK are (180 - 70) / 2, or 55 degrees each.

Angle GKJ must be 180 - (70 + 28) = 82.

Angle GKH must therefore be 82 - 55 = 27 degrees. Since two of the angles are 27 and 28 degrees, and the third is 180 - 55 = 125, the triangle is scalene.

36. As shown on the set of axes below, △GHS has vertices G(3,1), H(5,3), and S(1,4). Graph and state the coordinates of △G″H″S″, the image of △GHS after the transformation T_−3,1 ∘ D₂.

Answer:

If a Composition of Transformations, you go from RIGHT TO LEFT. You want the Translation OF THE Dilation, not a tranlation followed by a dilation.

Make sure you state the coordinates. Don't just label them.

37. In the diagram below, △ABC ∼ △DEF, DE = 4, AB = x, AC = x + 2, and DF = x + 6.

Determine the length of AB. [Only an algebraic solution can receive full credit.]

Answer:

If the triangles are similar then their sides are proportional. Set up a proportion and solve the quadratic equation that results from it.

x / (x + 2) = 4 / (x + 6)

x(x + 6) = 4(x + 2)

x² + 6x = 4x + 8

x² + 2x - 8 = 0

(x + 4)(x - 2) = 0

x = -4 (discard) or x = 2

Side AB has length = 2.

Check: 2 / 4 = 4 / 8 (check!)

Part IV: A correct answer will receive 6 credits. Partial credit is available.


38. Given: △ABC with vertices A(−6,−2), B(2,8), and C(6,−2)

AB has midpoint D, BC has midpoint E, and AC has midpoint F

Prove: ADEF is a parallelogram

ADEF is not a rhombus

[The use of the grid below is optional.]

Answer:

The fastest way to show that ADEF is a parallelogram is to find the slopes of the opposite sides.

The fastest way to show that ADEF is NOT a rhombus is to find the slopes of the diagonals. This is likely quicker than using the distance formula for consecutive sides, which is an alternate method.

First, find the midpoints:

D = ( (-6+2)/2, (-2+8)/2) = (-2,3)

E = ( (2+6)/2, (8+-2)/2) = (4,3)

F = ( (-6+6)/2, (-2+-2)/2) = (0,-2)

Slope of AD = (-2 - 3) / (-6 - -2) = -5/-4 = 5/4

Slope of EF = (-2 - 3) / (0 - 4) = -5/-4 = 5/4

Slope of DE = 0 (same y-coordinate)

Slope of AF = 0 (same y-coordinate)

ADEF is a parallelogram because the opposite sides are parallel.

Slope of AE = (-2 - 3) / (-6 - 4) = -5/-10 = 1/2

Slope of DF = (-2 - 3) / (0 - -2) = -5/2

The product of (1/2)(-5/2) = (-5/4) =/= -1.

ADEF is not a rhombus because the diagonals are not perpendicular.

or

Because AF is horizontal, it is easy to calculate its length. AF = (0 - -6) = 6 (or count the boxes if you used the grid.)

The length of AD is SQRT( (-6 - -2)² + (-2 - 3)² )

= SQRT( (-4)² + (-5)² )

= SQRT (16 + 25) = SQRT (41) =/= 6

ADEF is not a rhombus because not all sides are congruent.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, August 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, August 2011

Part IV: Each correct answer will receive 4 credits. Partial credit is available.


37. Vince buys a box of candy that consists of six chocolate pieces, four fruit-flavored pieces, and two mint pieces. He selects three pieces of candy at random, without replacement.

Calculate the probability that the first piece selected will be fruit flavored and the other two will be mint.

Calculate the probability that all three pieces selected will be the same type of candy.

Answer:

There is a total of 12 pieces of candy. You are selecting without replacement. That means that the number of items left will decrease.

Part 1: P(fruit then mint then mint), order matters:

= 4/12 * 2/11 * 1/10 = 8/1320

Part 2: P(all the same) = P(chocolate, chocolate, chocolate) + P(fruit, fruit, fruit). Note that the probability of 3 mint is zero because there are only two mint.

= 6/12 * 5/11 * 4/10 + 4/12 * 3/11 * 2/10

= 120 / 1320 + 24 / 130

= 144 / 1320

These can be reduced to 1/165 and 6/55, respectively, but isn't necessary. In probability, it is useful to have the same denominator for easy comparisons.

38. 8 On the set of axes below, solve the following system of equations graphically and state the coordinates of all points in the solution set.

y = −x² + 6x − 3
x + y = 7

Answer:

If you are using a graphing calculator, rewrite x + y = 7 as y = -x + 7. If you are not using one, then you know that it's in Standard Form and the x-intercept and the y-intercept are both 7.

If you are plugging in numbers, you know that the vertex will be when x = -b/(2a) = -(6)/(2(-1)) = 3.

The vertex, therefore, is y = -(3)² + 6(3) - 3 = 6, (3, 6).

Don't forget to lavel the lines and the points of intersection (which are the solutions).

39. Solve for m: m / 5 + 3(m - 1) / 2 = 2(m - 3)

Answer:

If you multiply the equation by 10 (or by (5) and (2)), you can remove the denominators from the equation.

(10) ( m / 5 + 3(m - 1) / 2 ) = 2(m - 3) (10)

2m + 15m - 15 = 20m - 60

17m - 15 = 20m - 60

45 = 3m

m = 15

Check: 15 / 5 + 3(15 - 1) / 2 ?= 2(15 - 3)
3 + 3(14)/2 ?= 2(12)
3 + 21 = 24
CHECK!

End of Part Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Peace

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

And Good Will, too. It wasn't a question.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, August 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, August 2011

Part II: Each correct answer will receive 2 credits. Partial credit is available.


32. Write an equation of the circle graphed in the diagram below.

Answer:

The equation of a circle is given by the equation (x - h)² + (y - k)² = r², where (h,k) is the center of the circle and r is the radius.

The center of the circle is (5,-4). The radius is 6. (Count from the center to the top or the bottom.)

So the equation is (x - 5)² + (y + 4)² = 36</P>

33. The diagram below shows △ABC, with AEB, ADC, and ∠ACB ≅ ∠AED. Prove that △ABC is similar to △ADE.

Answer:

Two triangles are similar if they have two angles that are congruent. (The third will be automaticallys congruent.)

It is given that ∠ACB ≅ ∠AED, and ∠A ≅ ∠A by the Reflexive property. So △ABC is similar to △ADE by the AA Similarity Theorem.

34. Triangle ABC has vertices A(3,3), B(7,9), and C(11,3). Determine the point of intersection of the medians, and state its coordinates. [The use of the set of axes below is optional.]

Answer:

The use of the set of axes below is optional but this is one time that it is probably better than trying to find the answer algebraically.

Graph the triangle. Then graph the medians from each vertex to the middle of the opposite side. You will only need to draw two of them, but draw the third one as a check.

The point of intersection (point of concurrence) is (7,5).

This one isn't too difficult when you notice that AC is a horizontal line, and its midpoint is (7,3). This means that the median from B to AC is a vertical line with a length of 6. The cetroid will be 2/3 of the way from B to AC, which is 4 units below (7,9). That would be (7,5).

This might have been more complicated if it were a Part IV question.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.