August 2022 Geometry Regents, Part II

This exam was adminstered in August 2022. These answers were not posted until they were unlocked on the NY Regents website or were posted elsewhere on the web.

August 2022 Geometry, Part II

Each correct answer is worth up to 2 credits. Partial credit can be given. Work must be shown or explained.

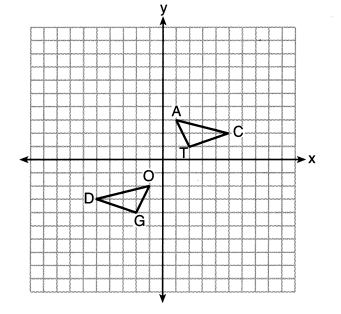
25. On the set of axes below, △DOG ≅ △CAT

Describe a sequence of transformations that maps △DOG onto △CAT.

Answer:
There are a few possible answers.

First, a reflection over the y-axis follow by translation 5 units up. Second, you could do that in the opposite order.

It is possible to reflect over different vertical or horizontal lines and then translate the image until it maps onto CAT.

A rotation would not work. The image would not be oriented correctly.

26. In right triangle MTH shown below, m∠H = 90°, HT = 8, and HM = 5.

Determine and state, to the nearest tenth, the volume of the three-dimensional solid formed by rotating △MTH continuously around MH.

Answer:
The three-dimensional solid will be a cone. The volume of a cone is given by the formula V = 1/3 πr²h.

V = 1/3 πr²h = 1/3 π (8)² (5) = 335.10...

V = 335.1

27. Using a compass and straightedge, dilate triangle ABC by a scale factor of 2 centered at C.
[Leave all construction marks.]

Answer:
This has to be one of the simplest construction problems that I have ever seen used.

Using the straightedge, extend line CA past A and extend line CB past B.

Use the compass and measure CA. Move to point A and make a mark on the extended line that is the same length.

Repeat this for CB.

Use the straightedge to draw the third side of the triangle.

You could also draw a circle around A with radius AC and a circle around B with radius BC.

28. A rock-climbing wall at a local park has a right triangular section that slants toward the climber, as shown in the picture below. The height of the wall is 5 meters and the slanted section begins 1.2 meters up the wall at an angle of 14 degrees.

Determine and state, to the nearest hundredth, the number of meters in the length of the section of the wall that is slanted (hypotenuse).

Answer:
This questions seems more complicated that it actually is. Label the photo and you will see that the leg of the triangle is 3.8, because it is 5 - 1.2. They want the hypotenuse and they have given you the side adjacent to the angle. That means you need to use Cosine.

Cos 14 = 3.8 / x
x = 3.8 / Cos 14 = 3.916... = 3.92.

29. In the diagram below of right triangle BAL, altitude AD is drawn to hypotenuse. The length of AD is 6.

If the length of DL is four times the length of BD, determine and state the length of BD.

Answer:
The Right Triangle Altitude Theorem says that (AD)² = (BD)(DL), and we know that DL = 4(BD).

6² = x(4x)
4x² = 36
x² = 9
x = 3

BD = 3.

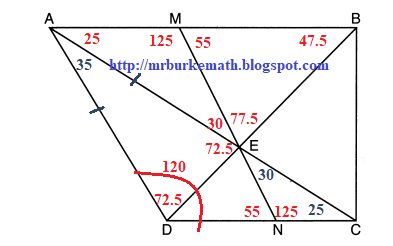
30. Trapezoid ABCD, where AB || CD, is shown below. Diagonals AC and DB intersect MN at E, and AD ≅ AE.

If m∠DAE = 35°, m∠DCE = 25°, and m∠NEC = 30°, determine and state m∠ABD.

Answer:
Label what you know and calculate angles until you get to ABD. Since AD ≅ AE, then m∠ADE = m∠AED = 72.5. Vertical angles are congruent. Alternate interior angles are congruent. The sum of the angles of a triangle is 180.

Look at the image below:

m∠ABD = 47.5

31. In the diagram below of circle 0, the measure of inscribed angle ABC is 36° and the length of OA is 4 inches.

Determine and state, to the nearest tenth of a square inch, the area of the shaded sector.

Answer:
The inscribed angle is 36 degrees, so the central angle is twice as big, or 72 degrees.

The area of the sector of the circle is A = (72/360) πr².

A = (72/360) (3.141592) (4)² = 10.053... = 10.1

End of Part II

How did you do?

Questions, comments and corrections welcome.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

August 2022 Algebra 1 Regents, Part IV

This exam was adminstered in August 2022. These answers were not posted until they were unlocked on the NY Regents website or were posted elsewhere on the web.

More Regents problems.

August 2022

Part IV: A correct answer will receive 6 credits. Partial credit can be earned.


37. An ice cream shop sells small and large sundaes. One day, 30 small sundaes and 50 large sundaes were sold for $420. Another day, 15 small sundaes and 35 large sundaes were sold for $270. Sales tax is included in all prices.

If x is the cost of a small sundae and y is the cost of a large sundae, write a system of equations to represent this situation.

Peyton thinks that small sundaes cost $2.75 and large sundaes cost $6.75. Is Peyton correct? Justify your answer.

Using your equations, determine algebraically the cost of one small sundae and the cost of one large sundae.

Answer:

In the first part, translate the sentences in the problem into a system of equations. In the second part, substitute 2.75 and 6.75 into the system of equations and see if you get two true statements. Chances are that Peyton is wrong because the third part wants you to solve the system of equations. (This fact is NOT proof. You still need to justify your work.)

Part 1:

30x + 50y = 420
15x + 35y = 270

Part 2:

30(2.75) + 50(6.75) = 420
15(2.75) + 35(6.75) = 277.50 =/= 270

Peyton is incorrect. His values do not work for the second day.

30x + 50y = 420
15x + 35y = 270

30x + 50y = 420
-30x - 70y = -540

-20y = -120

y = 6.00

30x + 50(6) = 420
30x + 300 = 420
30x = 120
x = 4.00

A small sundae is $4.00 and a large sundae is $6.00.

End of Exam

How did you do?

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

August 2022 Algebra 1 Regents, Part III

This exam was adminstered in August 2022. These answers were not posted until they were unlocked on the NY Regents website or were posted elsewhere on the web.

More Regents problems.

August 2022

Part III: Each correct answer will receive 4 credits. Partial credit can be earned. One mistake computational will lose 1 point. A conceptual error will lost half credit, or 2 points. It is sometimes possible to get 1 point for a correct answer with no correct work shown.


33. Thomas took a 140-mile bus trip to visit his grandparents. His trip is outlined on the graph below.

Explain what might have happened in the interval between D and E

State how many miles per hour the bus was traveling during this interval.

What was the average rate of speed, in miles per hour, for Thomas's entire bus trip?

Answer:

Between D and E, the distance was unchanged. The bus might have stopped moving for 1/2 hour.

The interval where the bus traveled the fastest would have the steepest slope. The slope of AB is 20/.5 = 40. The slope of CD is 90/1.5 = 60. The slope of EF is 30/1 = 30. Between C and D the bus went the fastest.

The bus was moving at 60 mph during this time. (Note: if you just counted boxes to find the steepest slope, then you need to work it out here.)

The entire trip was 140 miles in 4 hours, and 140/4 = 35 miles per hour.

34. Graph f(x) and g(x) on the set of axes below.

f(x) = x² - 4x + 3
g(x) = 1/2 x + 1

Based on your graph, state one value of x that satisfies f(x) = g(x). Explain your reasoning.

Answer:

Graph and label both equations.

One point of intersection is (4,3). The point is on both lines, so it is a solution to both equations. So for x = 4, f(4) = g(4) = 3.

Make sure you state the value of x. Don't just give the intersection point. This sounds silly, but it's important. It's what the question asked.

The other point looks like x = 1/2, but unless you solve it algebraically, or check it in the calculator, don't assume that it is just from eyeballing the graph. That said, x = 1/2 is, in fact, the other solution.

35. A store sells grapes for $1.99 per pound, strawberries for $2.50 per pound, and pineapples for $2.99 each. Jonathan has $25 to buy fruit.

He plans to buy 2 more pounds of strawberries than grapes. He also plans to buy 2 pineapples.

If x represents the number of pounds of grapes, write an inequality in one variable that models this scenario.

Determine algebraically the maximum number of whole pounds of grapes he can buy.

Answer:

Let x = # of pounds of graphs, and 1.99x = the cost of x pounds of grapes. Let x + 2 get the number of pounds of strawberries he buys, and 2.50(x + 2) = the cost of x + 2 pounds of strawberries. He cannot spend more than $25.

1.99x + 2.50(x + 2) + 2(2.99) < 25.00

1.99x + 2.50x + 5.00 + 5.98 < 25.00

4.49x + 10.98 < 25.00

4.49x < 14.02

x < 3.12...

The maximum number of whole pounds of grapes he can buy is 3.



36. Solve the system of inequalities graphically on the set of axes below.

Label the solution set S.

y + 3x < 5
1 > 2x - y
Is the point (-5,0) in the solution set? Explain your answer.

Answer:

First, rewrite these inequalities so that they are more useful. That could be Standard Form, if you like, or Slope-Intercept. The latter can be put into a calculator.

y + 3x < 5 can be rewritten as y < -3x + 5.

1 > 2x - y is the same as y > 2x - 1. (Add y to each side, then subtract 1 from each side.)

Draw and label the lines.

Remember that "<" means a dotted, dashed or broken line and you must shaded under the line. The line itself is NOT part of the solution.

The > means use a solid line and shade above the line. A solid line IS part of the solution set.

Make a large "S" in the section of the graph that is shaded twice.

Your graph should look similar to this:

The point (-5, 0) is in the solution set because it's in the section with the "S".

Note 1: If your graph is incorrect, answer according to what is on your graph if, for example, (-5,0) is not in the section shaded twice, you would say "No".

Note 2: If you didn't draw the graph, you could still answer this last part algebraically for a point. Solving counts as justification. You must have justification -- "Yes" or "No" by itself will NOT get any credit. If you substitute (-5,0) into each inequality, you will have two true statements, which means that it is a solution to the system.

End of Part III

How did you do?

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

August 2022 Algebra 1 Regents, Part II

This exam was adminstered in August 2022. These answers were not posted until they were unlocked on the NY Regents website or were posted elsewhere on the web.

More Regents problems.

August 2022

Part II: Each correct answer will receive 2 credits. Partial credit can be earned. One mistake (computational or conceptual) will lose 1 point. A second mistake will lose the other point. It is sometimes possible to get 1 point for a correct answer with no correct work shown.


25. Graph f(x) = |x + 1| on the set of axes below.

Answer:

You can put this in your calculator, but this is a fairly simple one. An absolute value funtion written in the form f(x) = a|x - h| + k will have a vertex (h,k).

In this example, a = 1, so the left side of the graph will have a slope of -1 and the right side will have a slope of 1. (h,k) is (-1,0) because there is a plus sign instead of a minus, and there is no k.

The graph will look like this:

26. The table below shows the value of a particular car over time.

Determine whether a linear or exponential function is more appropriate for modeling this data. Explain your choice.

Answer:

A linear function would be decreasing by approximately the same amount of money over time. An exponential function would be decreasing by approximately the same percentage or ratio over time.

10550 / 20000 = 0.5275, 5570 / 10550 = 0.52796..., 2940 / 5570 = 0.5278...

10550 - 20000 = -9450, 5570 - 10550 = -4980, 2940 - 5570 = -2630

An exponentional function is more appropriate because the value decreases to about 53% of the prior value every five years. That is, it is decreasing by 100% - 53% = 47%.

27. Is the product of √(8) and √(98) rational or irrational? Justify your answer.

Answer:

You can multiply and take the square root, or you can use factor trees.

√(8) * √(98) = √(784) = 28, which is a Rational number.

√(8 * 98) = √(2 * 2 * 2 * 2 * 7 * 7) = √( (2 * 2) * (2 * 2) * (7 * 7) ) = 2 * 2 * 7 = 28, which is a Rational number.

28. The ages of the last 16 United States presidents on their first inauguration day are shown in the table below.

Determine the interquartile range for this set of data.

Answer:

If you put this data into a list in your calculator, make sure you write down the Five Number Summary as your "work" for the problem.

To find the median and the quartiles, the data must be first put into order.

43, 46, 47, 51, 51, 52, 54, 54, 55, 56, 60, 61, 62, 64, 69, 70

There are 16 pieces of data, so the median is between the 8th and 9th numbers, 54 and 55. The median is 54.5.

Q1 is between the 4th and 5th numbers, which are both 51, so Q1 = 51. Q3 is between the 12th and 13th numbers, which are 61 and 62, so Q3 = 61.5.

IQR = Q3 - Q1 = 61.5 - 51 = 10.5.

29. The cost of one pound of grapes, g, is 15 cents more than one pound of apples, a.

The cost of one pound of bananas, b, is twice as much as one pound of grapes.

Write an equation that represents the cost of one pound of bananas in terms of the cost of one pound of apples.

Answer:

Rewrite the sentences as equations. The word "is" is your equal sign.

g = 15 + a
b = 2g

So b = 2(a + 15) = 2a + 30

Either of those two expressions should be acceptable after "b =".

30. A student is given the functions f(x) = (x + 1)² and g(x) = (x + 3)².

Describe the transformation that maps f(x) onto g(x).

Answer:

The +1 moves (translates) the function 1 unit to the left of the parent function (not listed), and the +3 moves the function 3 units to the left of the parent function.

So transformation that maps f(x) onto g(x) is a translation two units to the left.

31. Solve 3x² - 5x - 7 = 0 algebraically for all values of x, rounding to the nearest tenth.

Answer:

"To the nearest tenth" tells you that it's not likely to be an integer and could be an irrational answer. If that is the case, do not answer in radical form. Convert it to a decimal and evaluate.

If you multiply 3*(-7), you get -21. There are no factors of -21 with a sum of -5, so you need to use the Quadratic Formula. See below:

x = 5/6 + &radic(109)/6 = 2.57... or x = 5/6 - &radic(109)/6 = -0.90...

x = 2.6 or x = -0.9

32. Factor completely: 3y² - 12y - 288

Answer:

The words "factor completely" usually mean that there will be more than one step. When you are finished, look again to make sure you actually are finished.

3y² - 12y - 288

3(y² - 4y - 96)

3(y + 8)(y - 12)

End of Part II

How did you do?

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, August 2010)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, August 2010

Part IV: A correct answer will receive 6 credits. Partial credit is available.


38. Given: Quadrilateral ABCD has vertices A(−5,6), B(6,6), C(8,−3), and D(−3,−3).
Prove: Quadrilateral ABCD is a parallelogram but is neither a rhombus nor a rectangle.
[The use of the grid below is optional.]

Answer:

You don't need to graph the parallelogram, but if you do it will be "obvious" that it is neither a rectangle nor a rhombus. However, despite it being "obvious", you still have to PROVE it. (Seriously, writing "Look! It is isn't!" might get a laugh from the scorer, but no credit at all.) To show that ABCD is a a parallelogram, show that AB || CD and AD || BC. You can do this by finding the slopes of all four sides.

To show that ABCD is NOT a rectangle, show that two consecutive sides are not perpendicular -- you already have the slopes of the sides, so this is trivial.

To show that ABCD is NOT a rhombus, either show that two consecutive sides are not congruent, that is, they have different lengths, OR show that the slopes of the diagonals AC and BD are not perpendicular. Either is acceptable.

Slope of AB = (6-6)/(6-(-5)) = 0
Slope of BC = (-3-6)/(8-6) = -9/2
Slope of CD = (-3-(-3))/(-3-8) = 0
Slope of DA = (6-(-3))/(-5-(-3) = 9/(-2) = -9/2

The opposite sides have the same slopes, so the opposite sides are parallel. Since the opposite sides are parallel, the quadrilateral is a parallelogram.

Slope of AC = (-3 - 6)/(8 - (-5)) = -9/13
Slope of BD = (-3 - 6)/(-3 - 6) = -9/-9 = 1

Since AC is not perpendicular BD, then the diagonals of the parallelogram are not perpendicular, so the quadrilateral cannot be a rhombus.

Length of AB = √((-5-6)² + (6-6)²) = -11
Length of BC = √((6-8)² + (6-(-3))²) = √(4 + 81) = √(85)

The consecutive sides of the quadrilateral are not congruent, so the parallelogram cannot be a rhombus.

End of exam. How did you do?

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Order the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, August 2010)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, August 2010

Part IV: Each correct answer will receive 4 credits. Partial credit is available. One computational error will cost 1 credit. A conceptual error will usually cost 2 credits.


37. On the set of axes below, solve the following system of inequalities graphically.

y < 2x + 1
y ≥ −1/3 x + 4

State the coordinates of a point in the solution set.

Answer:

The inequalities are already in slope-intercept form, making them easy to graph without a calculator or even a table of values. (You can make a table of values if you want to.)

A "less than" sign means that the line will be broken/dashed, and the shading will go beneath the line. Any points on that line are NOT points in the solution set.

A "greater than or equal to" sign means that the line will be solid, and the shading will go above the line. Any points on that line are solutions to this inequality, but not necessarily to the entire system.

The solution set to the system is the section of the graph that is shaded twice.

Pick any point in the double-shaded area, such as: (5,5).

38. Each of the hats shown below has colored marbles placed inside. Hat A contains five green marbles and four red marbles. Hat B contains six blue marbles and five red marbles. Hat C contains five green marbles and five blue marbles.

If a student were to randomly pick one marble from each of these three hats, determine from which hat the student would most likely pick a green marble. Justify your answer.

Determine the fewest number of marbles, if any, and the color of these marbles that could be added to each hat so that the probability of picking a green marble will be one-half in each of the three hats.

Answer:

For the first part, you have to determine P(green) for each hat. Then state which hat has the greatest P(green). (Note that there are NO green marbles in Hat B.) For the second part, if any hat has less P(green) < 1/2, how many green marbles need to be added to make the probability equal 1/2. If any hat has P(green) > 1/2, how many marbles of a different color would need to be added.

Hat A: P(Green) = 5 / (5+4) = 5/9

Hat B: P(Green) = 0. (There are no green marbles in Hat B.)

Hat C: P(Green) = 5 / (5+5) = 5/10 = 1/2.

Hat A has the greatest probability of selecting a green marble.

Part 2:

Hat A: Add 1 red (or any non-green) marble and there will be 5 green and 5 red. P(Green) = 1/2.

Hat B: There are 11 marbles in the hat. Add 11 green marbles and P(Green) = 11/22 = 1/2.

Hat C: Do not add any marbles.

39. A hot-air balloon is tied to the ground with two taut (straight) ropes, as shown in the diagram below. One rope is directly under the balloon and makes a right angle with the ground. The other rope forms an angle of 50° with the ground.

Determine the height, to the nearest foot, of the balloon directly above the ground/

Determine the distance, to the nearest foot, on the ground between the two ropes.

Answer:

The height above the ground is the side opposite the angle. You have the length of the rope, with is the hypotenuse of the triangle. Therefore, to find the height, you need to use the Sine ration.

To find the distance on the ground, you have three choices: you can use the cosine ration, the tangent ratio, or Pythagorean Theorem.

Height: sin 50 = x / 110

x = 110 sin 50 = 84.26..., or 84 feet.

Distance on the ground:

a) cos 50 = x / 110
x = 110 cos 50 = 70.70..., or 71 feet.

b) tan 50 = 110 / x
x = 84 / tan 50 = 70.48..., or 70 feet -- If you did this, they would have to accept your answer because you used the answer from part 1, rounded the way that you were told to.
(I am not 100% certain of that, but some scorer should've been arguing in your favor.)

c) x² + 84² = 110²
x² + 7056 = 12100
x² = 5044
x = 71.02..., or 71 feet.

End of Part Exam. How did you do?

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Order the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Only Numbers

(Click on the comic if you can't see the full image.)

(C)Copyright 2022, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

Letters posing as numbers? Nothing is what it seems to be!

I was originally going to use variables and call the building the "Algebraica" but then simplified it. Since pi is introduced in early grades as a number, I went with e and i. R was an afterthought because I thought I needed something else and I didn't want to use another alphabet.

The font that the show uses is called The New Yorker (like the magazine). That and several similar fonts were available for download, but only for personal use. Right now, this website is personal, but I can't say that this will always be the case. And, technically, since Google ads have been turned on, this hobby could be considered a business by people who wish to bust your chops and take your money. So I went generic.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, August 2010)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, August 2010

Part III: Each correct answer will receive 4 credits. Partial credit is available.


35. In the diagram below of quadrilateral ABCD with diagonal BD, m∠A = 93, m∠ADB = 43, m∠C = 3x + 5, m∠BDC = x + 19, and m∠DBC = 2x + 6. Determine if AB is parallel to DC. Explain your reasoning.

Answer:

If m∠ABD = x + 19 then the two alternate interior angles will be equal and the lines must be parallel. So naturally, you have a lot of work to do to find the size of both of those angles.

To find m∠ABD, notice that you have two of the three angles in the triangle, which has a sum of 180 degrees. In triangle BCD, you have to solve 2x + 6 + 3x + 5 + x + 19 = 180 for x and then evaluate x + 19.

m∠ABD + 43 + 93 = 180
m∠ABD + 136 = 180
m∠ABD = 44

2x + 6 + 3x + 5 + x + 19 = 180
6x + 30 = 180
6x = 150
x = 25

x + 19 = 25 + 19 = 44

Because the alternate interior angles are congruent, line AB and CD must be parallel.

36. The coordinates of the vertices of △ABC are A(1,3), B(−2,2), and C(0,−2). On the grid below, graph and label △A″B″C″, the result of the composite transformation D₂ ∘ T_3,−2. State the coordinates of A″, B″, and C″.

Answer:

The most important thing to remember here is that D₂ ∘ T_3,−2 means "the dilation of the translation". That means that you must do the translation FIRST and then do the dilation.

You must graph this for full credit. However, algebraically, these are the points:

A(1,3) -> A'(1+3,3-2) = A'(4,1) -> A"(4*2,1*2) = A"(8,2)

B(-2,2) -> B'(-2+3,2-2) = B'(1,0) -> B"(1*2,0*2) = B"(2,0)

C(0,-2) -> C'(0+3,-2-2) = C'(3,-4) -> C"(3*2,-4*2) = C"(6,-8)

You graph should look like the following. The points MUST be labels. The coordinates can be written on the graph or on the side of the graph. For instance, if three lines above are written on your paper, than just A", B" and C" are sufficient on the graph itself.

You may get credit if you get A'B'C' correct but do not finish correctly. However, A'B'C' is not required for full credit. It's just a good idea to include it.

37. In the diagram below, △RST is a 3-4-5 right triangle. The altitude, h, to the hypotenuse has been drawn. Determine the length of h.

Answer:

Label the point where the altitude intersects RS as point U. There are three right triangles: RST, RTU, and STU. These three triangles are similar and their corresponding sides are proportional.

We can find the length of b by comparing short/hypotenuse in RTU and RST:

b/3 = 3/5

5b = 9

b = 9/5

This means that a = 5 - b = 5 - 9/5 = 25/5 - 9/5 = 16/5.

The Right Triangle Altitude Theorem tells us that h² = (b)(a).

h² = (9/5)(16/5) = 144/25

h = 12/5

You could also have had the decimal equivalents a = 3.2, b = 1.8, and h = 2.4.

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Order the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, August 2010)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, August 2010

Part III: Each correct answer will receive 3 credits. Partial credit is available. One computational error will cost 1 credit. A conceptual error will usually cost 2 credits.


34. The number of songs fifteen students have on their MP3 players is:

120, 124, 132, 145, 200, 255, 260, 292, 308, 314, 342, 407, 421, 435, 452

State the values of the minimum, 1st quartile, median, 3rd quartile, and maximum.

Using these values, construct a box-and-whisker plot using an appropriate scale on the line below.

Answer:

The numbers are already in order, so the first step is down for you. Next, you need to find the Five-Number Summary. There are so few pieces of data that it will be quicker to do by hand than if you put it in your calculator. (If you use your calculator, double-check your data entry.)

There are 15 pieces of data. The first, 120, is the Minimum. The last, 452, is the Maximum. The number in the middle of the 15 is the 8th piece of data, is the Median, 292.

There are 7 pieces of data less than the median and 7 more that are greater than the median. The middle number of the lower 7, the fourth number, is the First Quartile (Q1), 145. The middle number of the upper 7, the fourth from the end, or the 11th, is the Third Quartile (Q3), 407.

You can now make the box-and-whisker plot above the number line. Before you do this, you have to decide upon a scale to use to number it. The Range = Maximum - Minimum = 452 - 120 = 332. Since there are only 21 tick marks on the number line, you need to use a scale of 20 or larger. Don't make it too large, or you wrote be able to plot the points accurately.

DO NOT DRAW THE BOX AND WHISPER PLOT AND THEN NUMBER THE LINE. YOU WILL LIKELY LOSE 2 OUT OF THE 3 POINTS.

Look at the image below:

35. Find the volume, in cubic centimeters, and the surface area, in square centimeters, of the rectangular prism shown below.

Answer:

The volume of a rectangular prism is the product of the Length times the Width times the Height. The surface area is the total area of the six rectangles: the front and back, the left and right, and the top and bottom. Each pair listed in the previous sentence are congruent, so you find one and double it.

V = 10 * 2 * 4 = 80 cm³.

S = 2(10)(2) + 2(10)(4) + 2(2)(4) = 136 cm².

SHOW YOUR SUBSTITUTIONS. Don't just put the numbers in the calculator and write the answers.

You don't need to include the units because they are in the question. HOWEVER, if you include the units, and you make a mistake, such as writing cm² for Volume, you will lose a point.

36. Find the roots of the equation x² = 30 − 13x algebraically.

Answer:

Algebraically means that if you do it some other way, like sketching a graph or using your calculator, you will not get full credit. You would likely get only 1 credit out of the 3.

That said, if you make a graph, you will know the answer that you need to find when you do it algebraically, and you'll know if you've made a mistake.

x² = 30 − 13x

x² + 13x - 30 = 0

(x + 15)(x - 2) = 0

x + 15 = 0 or x - 2 = 0

x = -15 or x = 2

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Order the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, August 2010)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, August 2010

Part II: Each correct answer will receive 2 credits. Partial credit is available. One computational or conceptual error will cost 1 credit. A second will cost the other.


32. On the line segment below, use a compass and straightedge to construct equilateral triangle ABC. [Leave all construction marks.]

Answer:

Place the point of the compass on point A and open it up until the pencil is on point B.

Make an arc above the line. Without changing the size of the compass, turn the compass around and make a second arc from point A. Make a point where the arcs intersect. Use the straightedge to draw the triangle.

Look at the image below. Do not erase any of the marks. Do not add any others. If you don't use the compass or the straightedge, it will be obvious that you did not and you will lose credit.

33. AIn the diagram below, car A is parked 7 miles from car B. Sketch the points that are 4 miles from car A and sketch the points that are 4 miles from car B. Label with an X all points that satisfy both conditions.

Answer:

All the points that are 4 miles from point A is a circle with point A at the center. Likewise, you need to draw a circle around point B.

Because the points are only 7 miles apart, the two radii will overlap because 4 + 4 = 8, which is more than 7.

Your "sketch" doesn't have to be perfect but your circles must intersect at two points in between A and B. The circles should be about the same size, the best that you can draw them that way. (Again, it doesn't have to be perfect.) Points A and B should not be together inside either circle.

34. Write an equation for circle O shown on the graph below.

Answer:

The equation for a circle is (x - h)² + (y - k)² = r², where (h,k) is the center of the circle, and r is the radius. Notice that there are minus signs in the equation.

The center of the circle is (-1,2) and the radius is 6. (Start at point O and count the boxes until you hit the circle.)

The equation is (x - (-1))² + (y - 2)² = 6²

which is (x + 1)² + (y - 2)² = 36

End of Part II.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Order the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.