(x, why?): April 2022

Friday, April 29, 2022

One-Off Topics

(Click on the comic if you can't see the full image.)

Like the compass and straightedge you pull out for that one lesson before you complain about how lousy the compasses are these days.

Inspiration for this came from my cropping a group of students from a "characters" file to include in the backgound of a slide in one of my classes. Then it only made sense to include the perennial substitute, Quinn Jonas.

The tricky parts were deciding what topic to mention (before realizing that I didn't actually have to mention one), and what I was going to title this strip.

Speaking of titles and names of things: any suggestions for these guys? The one with the X across his shirt might have a name, although possibly not canonical.

I also write Fiction!

You can now ~~pre~~order Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below.
Preorder the softcover or ebook at Amazon.

Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides.
Available in softcover or ebook at Amazon.

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Tuesday, April 26, 2022

Algebra Problems of the Day (Integrated Algebra Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, January 2011

Part IV: Each correct answer will receive 4 credits. Partial credit is available.

37. An oil company distributes oil in a metal can shaped like a cylinder that has an actual radius of 5.1 cm and a height of 15.1 cm. A worker incorrectly measured the radius as 5 cm and the height as 15 cm.

Determine the relative error in calculating the surface area, to the nearest thousandth.

Answer:

Remember that they are asking for relative error, which is a decimal, not the percent of error. If you give the answer as a percentage, you will lose a point. (No, I don't agree with that, but I don't write the rubric.)

The relative error is the difference between the actual amount and the measured amount, divided by the actual amount.

You don't actually have to find the two surface areas, but you need to have the factors in your equation. Why would you do this? Because you can eliminate pi from the equation.

Surface area of a cylinder is equal to 2 π r² + 2 π r h

( (2 π (5.1)² + 2 π (5.1)(15.1)) - (2 π (5)² + 2 π (5)(15) )
(2 π (5.1)² + 2 π (5.1)(15.1))

2π( ((5.1)² + (5.1)(15.1)) - ((5)² + (5)(15) )
((2 π)((5.1)² + (5.1)(15.1)))

( ((5.1)² + (5.1)(15.1)) - ((5)² + (5)(15) )
((5.1)² + (5.1)(15.1))

( 103.02 - 100 )
103.02

3.02
103.02

Which is approximately equal to 0.029 to the nearest thousandth.

38. The Booster Club raised $30,000 for a sports fund. No more money will be placed into the fund.
Each year the fund will decrease by 5%. Determine the amount of money, to the nearest cent, that will be left in the sports fund after 4 years.

Answer:

You can use the equation for exponential decay and get it in one shot, or you can you the calculate 5% of the total, subtract it and then repeat that three more times. You will get the same answer.

Do the long one if you don't remember how to do the quick method.

A = 30000(1.00 - 0.05)⁴ = 24435.19, to the nearest cent.

The longer way:

100 - 5% = 95% that remains, which is .95.

30000 * 0.95 = 28500

28500 * 0.95 = 27075

27075 * 0.95 = 25721.25

25721.25 * 0.95 = 24435.1875 = 24435.19

Note: According to the Answer Key, a result of 24435.20 was also acceptable because different models and brands of calculators yield slightly different results.

39. Graph the following system of inequalities on the set of axes shown below and label the solution set S.

y > −x + 2
y ≤ 2/3 x + 5

Answer:

Thankfully, the inequalities are already in slop-intercept form and are ready to be entered into your graphing calculator, if you have one.

Most that the first inequality says greater than. The line will be broken (dashed, dotted) and the graph will be shaded Above the line. The second inequality says less than or equal to. This line will be solid (part of the solution). The graph will be shaded below the line.

Make sure that you label the two lines, even if it looks "obvious" to you. Look at the graph below:

End of Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below.
Preorder the softcover or ebook at Amazon.

Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides.
Available in softcover or ebook at Amazon.

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Monday, April 25, 2022

Restful Sleep

(Click on the comic if you can't see the full image.)

If one wakes you up, the other will keep you up. Of course, one is more easier remedied than the other.

Age would be another factor.

I almost typed "fullness" on the bottom axis until it occured to me that I could use two different meanings for "volume".

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, January 2011

Part I: Each correct answer will receive 2 credits.

16. If x² + 2 = 6x is solved by completing the square, an intermediate step would be

1) (x + 3)² = 7
2) (x - 3)² = 7
3) (x - 3)² = 11
4) (x - 6)² = 34

Answer: 2) (x - 3)² = 7

First, 6x needs to be subtracted from both sides. To complete the square, you will end up with half of -6, which is -3. Eliminate Choice (1) and (4).

x² + 2 = 6x

x² - 6x + 2 = 0

x² - 6x + 9 + 2 = 0 + 9

x² - 6x + 9 = 9 - 2

(x - 3² = 7

This is Choice (2).

17. Three marbles are to be drawn at random, without replacement, from a bag containing 15 red marbles, 10 blue marbles, and 5 white marbles. Which expression can be used to calculate the probability of drawing 2 red marbles and 1 white marble from the bag?

1) ₁₅C₂ * ₅C₁ / ₃₀C₃
2) ₁₅P₂ * ₅P₁ / ₃₀C₃
3) ₁₅C₂ * ₅C₁ / ₃₀P₃
4) ₁₅P₂ * ₅P₁ / ₃₀P₃

Answer: 1) ₁₅C₂ * ₅C₁ / ₃₀C₃

This problem is a lot easier than it looks as soon as you see the choices. This is purely a Combination problem, not a permutation problem.

Therefore, the answer is Choice (1).

18. The expression x^(-2/5) is equivalent to

Answer: 4) [See image]

The negative exponent means that the x will move to the denominator. Eliminate Choices (1) and (2).

The denominator of 5 in the exponent means that the expression is the fifth root, which is Choice (4).

19. On January 1, a share of a certain stock cost $180. Each month thereafter, the cost of a share of this stock decreased by one-third. If x represents the time, in months, and y represents the cost of the stock, in dollars, which graph best represents the cost of a share over the following 5 months?

Answer: 3) [See image]

If something drops in 1/3 of its value every month, that is exponential decay. Eliminate Choices (1) and (2) which show linear graphs.

Choice (3) shows the stock losing 1/3 of its value. This is the correct response.

Choice (4) shows the stock reduced to 1/3 of its value. It has lost 2/3.

20. In the diagram below of right triangle JTM, JT = 12, JM = 6, and m∠JMT = 90

What is the value of cot J ?

1) √(3)/3
2) 2
3) √(3)
4) 2 √(3) / 3

Answer: 1) √(3)/3

You want cotangent, which means we need the adjacent over the opposite (the inverse of tangent). We know the adjacent is 6. We don't know the opposite, but we could find it.

If you realized that this is half of an equilateral traingle, and therefore a 30-60-90 right triangle, they you know that MT is JM time %radic;(3). If you didn't realize that, you can perform Pythagorean Theorem to get the same result.

So cot J = 6 / 6 %radic;(3) = 1 / %radic;(3) = 1 / %radic;(3) * %radic;(3) / %radic;(3) = %radic;(3) / 3, which is Choice (1).

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, January 2011)

More Regents problems.

Integrated Algebra Regents, January 2011

Part III: Each correct answer will receive 3 credits. Partial credit is available.

34. A line having a slope of 3/4 passes through the point (−8,4).

Write the equation of this line in slope-intercept form.

Answer:

You can write the equation in point-slope form and then convert it to slope-intercept form.

In point-slope form, it's either

y - 4 = 3/4(x + 8) or y = 3/4(x + 8) + 4

depending upon which way you learned it. If you wrote the first one, the first step is to add 4 to both sides and get the second one.

Then use the Distributive Property.

y = 3/4x + 6 + 4

y = 3/4x + 10

35. The test scores for 18 students in Ms. Mosher’s class are listed below:

86, 81, 79, 71, 58, 87, 52, 71, 87, 87, 93, 64, 94, 81, 76, 98, 94, 68
Complete the frequency table below:

Draw and label a frequency histogram on the grid below.

Answer:

Record a tally mark for each score in an interval. Then count them up to get the Frequency.

Next, use the table to make the histogram.

Things to take note of: the bars on your histogram need to be the same width, whatever that width is (2 boxes, 3 boxes); there needs to be a space on the x-axis and a squiggle to indicate a break because the x-axis is a number line and the bottom left corner is zero; each bar needs to be labeled with its interval; each of the axes needs to have a label; the histogram needs a title.

If any of those are missing or inconsistent, you can leave a point. Multiple mistakes will cost you two points from a three-point problem. That's a lot of credit to give up for silly mistakes after putting in all the effort.

36. Solve algebraically for x: (x + 2) / 6 = 3 / (x - 1)

Answer:

It's a proportion, so you can cross-multiply. Then solve the resulting quadratic equation by setting it equal to 0 and factoring.

(x + 2) / 6 = 3 / (x - 1)

(x + 2)(x - 1) = (3)(6)

x² + x - 2 = 18

x² + x - 20 = 0

(x + 5)(x - 4) = 0

x + 5 = 0 or x - 4 = 0

x = -5 or x = 4

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Sunday, April 24, 2022

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, January 2011

Part I: Each correct answer will receive 2 credits.

11. The conjugate of 7 − 5i is

1) −7 − 5i
2) −7 + 5i
3) 7 − 5i
4) 7 + 5i

Answer: 4) 7 + 5i

To find the conjugate of a complex number, change the sign of the imaginary part.

So the conjuage of 7 - 5i is 7 + 5i, which is Choice (4).

Similarly, the conjugate of the binomial (7x - 5y) is (7x + 5y).

12. If sin^-1 (5/8) = A, then

1) sin A = 5/8
2) sin A = 8/5
3) cos A = 5/8
4) cos A = 8/5

Answer: 1) sin A = 5/8

Remember that sin(sin^-1(5/8) = 5/8. Therefore,

sin^-1 (5/8) = A

sin( sin^-1 (5/8) ) = sin(A)

5/8 = sin A

That is Choice (1).

13. How many distinct triangles can be formed if m∠A = 35, a = 10, and b = 13?

1) 1
2) 2
3) 3
4) 0

Answer: 2) 2

You are given two sides and an angle that is not included between those two sides. We learned in Geometry that there is no SSA Theorem because there are two possible triangles in this situation.

The exception to this rule is the Hypotenuse Leg Theorem. This triangle is a right triangle if and only if the sin 35 = 10/13, so check this.

sin 35 degrees = 0.573...

10/13 = 0.7692...

They are not the same, so the triangle is not right.

14. When 3/2 x² − 1/4 x - 4 is subtracted from 5/2 x² - 3/4 x + 1, the difference is

1) -x² + 1/2 x - 5
2) x² - 1/2 x + 5
3) -x² - x - 3
4) x² - x - 3

Answer: 2) x² - 1/2 x + 5

The "from" term goes first in the subtraction:

(5/2 x² - 3/4 x + 1) - (3/2 x² − 1/4 x - 4)

= 5/2 x² - 3/2 x² - 3/4 x - (-1/4 x) + 1 - (-4)

= 2/2 x² - 2/4 x + 5

= x² - 1/2 x + 5, which is Choice (2).

Note that you would've gotten choice (1) if you subtracted backwards.

15. The solution set of the inequality x² − 3x > 10 is

1) {x | −2 < x < 5}
2) {x | 0 < x < 3}
3) {x | x < -2 or x > 5}
4) {x | x < -5 or x > 2}

Answer: 3) {x | x < -2 or x > 5}

Factor the inequality.

x² − 3x > 10

x² - 3x - 10 > 0

(x - 5)(x + 2) > 0

For the product to be positive, both binomials must be positive or both must be negative.

They are both positive is x > 5. They are both negative if x < -2. This is Choice (3).

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, January 2011)

More Regents problems.

Integrated Algebra Regents, January 2011

Part II: Each correct answer will receive 2 credits. Partial credit is available.

31. Roberta needs ribbon for a craft project. The ribbon sells for $3.75 per yard. Find the cost, in dollars, for 48 inches of the ribbon.

Answer:

Either convert 48 inches to yards, or convert $/yard to $/inch.

There are 36 inches in on 1 yard, so 48 inches is 48/36 or 4/3 yards.

(4/3)(3.75) = $5.00. Yes, you can multiply fractions and decimals.

Doing it the other way:

There are 36 inches in 1 yard, so ribbon sells for $3.75/36 per inch or 0.10416666... per inch. (You only need a few decimals to avoid the rounding error.

(0.104166666)(48) = 4.999999968, which rounds to $5.00.

32. The square dart board shown below has a side that measures 40 inches. The shaded portion in the center is a square whose side is 15 inches. A dart thrown at the board is equally likely to land on any point on the dartboard.

Find the probability that a dart hitting the board will not land in the shaded area.

Answer:

The area that isn't shaded is equal to th area of the entire board minus the area that is shaded.

Area of the board is 40² = 1600. The shaded area is 15² = 225.

The non-shaded area is 1600 - 225 = 1375.

So the probability that the dart will not land in the shaded area is 1375/1600. You can simplify it, but it isn't necessary. To simplify, divide by 25/25 and you'll get 55/64. If you make a mistake, or write a rounded decimal value, you will lose one of the two points, so be careful.

33. As shown in the diagram below, a ladder 5 feet long leans against a wall and makes an angle of 65° with the ground. Find, to the nearest tenth of a foot, the distance from the wall to the base of the ladder.

Answer:

The ladder forms the hypotenuse of the right triangle. The distance from the wall to the base of the ladder is the side adjacent to the angle. Adjacent and hypotenuse mean that we need to use the cosine.

cos 65^o = x / 5

x = 5 * cos 65^o = 2.113... or 2.1 feet.

If you got a negative answer, first you should realize that a negative distance is impossible. Second, you should realize that your calcluator is radians mode. Switch to to Degrees.

End of Part II.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2011

Part IV: A correct answer will receive 6 credits. Partial credit is possible.

35. Quadrilateral MATH has coordinates M(1,1), A(−2,5), T (3,5), and H(6,1). Prove that quadrilateral MATH is a rhombus and prove that it is not a square.
[The use of the grid on the next page is optional.]

Answer:

Graphing the points could help by giving you a visual reference point but it isn't necessary.

A rhombus has four congruent sides. You have show this to be true by using the distance formula four times.

Or you can show that the rhombus is a parallelogram by finding the slopes of the four sides and then finding the lengths of two consecutive sides with the distance formula. Why? Because finding slopes is easier as less likely to cause an arithmetic error.

HOWEVER, you can ALSO show that a quadrilateral is a rhombus if its diagonals are perpendicular to each other. This is actually the simplest method, but it is not the most obivous.

The slope of MT is (5-1)/(3-1) = 4/2 = 2. The slope of AH is (1-5)/(6-(-2)) = -4/8 = -1/2. MT is perpendicular to AH, so the quadrilateral is a rhombus.

To show that it is not a square, show that the sides of the rhombus are not perpendicular.

The slope of MA is (5-1)/(-2-1) = 4/-3. The slope of AT is (5-5)/(3-(-2)) = 0/5 = 0. The sides are not perpendiclar, so the rhombus is not a square.

Note that the second part would be obvious from a graph because two lines would be horizontal, but the other two lines would not be vertical.

If you use the distance formula for the first part, you would have found that:

MA = SQRT( (1-(-2)² + (1-5)² ) = SQRT(9 + 16) = 5

AT = SQRT( ((-2)-3² + (5-5)² ) = SQRT(25 + 0) = 5

TH = SQRT( (3-6)² + (5-1)² ) = SQRT(9 + 16) = 5

AT = SQRT( (6-1)² + (1-1)² ) = SQRT(25 + 0) = 5

All sides are congruent.

End of Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Saturday, April 23, 2022

Algebra Problems of the Day (Integrated Algebra Regents, January 2011)

More Regents problems.

Integrated Algebra Regents, January 2011

Part I: Each correct answer will receive 2 credits.

26. What is the result when 2x² + 3xy − 6 is subtracted from x₂ − 7xy + 2?

1) -x² - 10xy + 8
2) x² + 10xy − 8
3) -x² - 4xy − 4
4) x² - 3xy − 4

Answer: 1) -x² - 10xy + 8

The "from" goes on top and the other expression goes on the bottom.

x₂ − 7xy + 2
2x² + 3xy − 6

-x² - 10xy + 8

This is Choice (1).

Watch your signs when subtracting negatives.

27. What is an equation of the axis of symmetry of the parabola represented by y = −x² + 6x − 4?

1) x = 3
2) y = 3
3) x = 6
4) y = 6

Answer: 1) x = 3

The Axis of Symmetry is ALWAYS x = a number. Eliminate Choices (2) and (4).

The formula is x = -b/(2a), where a = -1 and b = 6.

So x = -(6)/(2(-1)) = -6/-2 = 3, which is Choice (1).

They didn't even try to trick you with x = -3 in case you forgot a sign.

28. Which equation has roots of −3 and 5?

1) x² + 2x - 15 = 0
2) x² - 2x - 15 = 0
3) x² + 2x + 15 = 0
4) x² -+ 2x + 15 = 0

Answer: 2) x² - 2x - 15 = 0

If the roots are -3 and 5, then multiply (x + 3)(x - 5).

(x + 3)(x - 5) = x² - 5x + 3x - 15 = x² - 2x - 15, which is Choice (2).

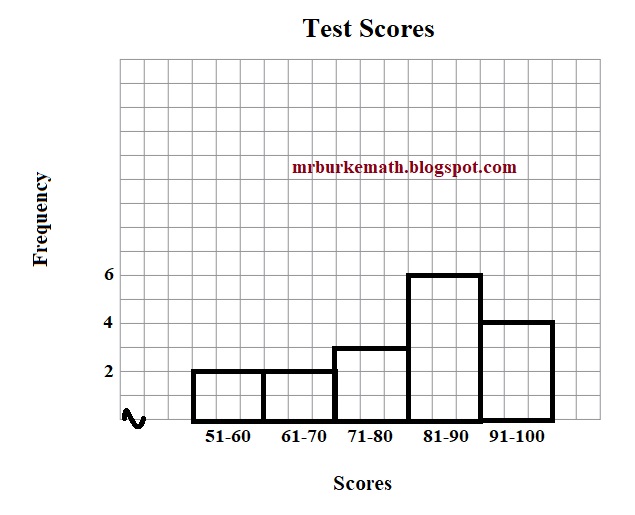
29. A spinner that is equally divided into eight numbered sectors is spun 20 times. The table below shows the number of times the arrow landed in each numbered sector.

Based on the table, what is the empirical probability that the spinner will land on a prime number on the next spin?

1) 9/20
2) 11/20
3) 12/20
4) 14/20

Answer: 3) 12/20

The prime numbers on the spinner are 2, 3, 5, and 7. (1, 4, 6, and 8 are NOT prime numbers.) Those four numbers appeared 3, 2, 4, and 3 times, respectively.

The sum of 3 + 2 + 4 + 3 = 12, so the empirical probability is 12/20, which is Choice (3).

30. Which expression represents (x² - x - 6) / (x² − 5x + 6) in simplest form?

1) (x + 2) / (x - 2)
2) (-x - 6) / (-5x + 6)
3) 1/5
4) 1

Answer: 1) (x + 2) / (x - 2)

Since the numerator does NOT match the denominator, it should be obvious that the answer is NOT 1. Eliminate Choice (4).

Factor both the numerator and the denominator into two binomials. Cancel out the common pairs which make 1.

(x² - x - 6) factors into (x - 3)(x + 2).

(x² − 5x + 6) factors into (x - 3)(x - 2)

(x - 3)/(x - 3) = 1

So the fraction reduces to (x + 2)/(x - 2), which is Choice (1).

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2011

Part III: Each correct answer will receive 4 credits. Partial credit is possible.

35. On the set of axes below, graph the locus of points that are four units from the point (2,1). On the same set of axes, graph the locus of points that are two units from the line x = 4. State the coordinates of all points that satisfy both conditions.

Answer:

The first locu of points is a circle with a radius of 4 and a center at (2,1). The second locus of points are two vertical lines, x = 2 and x = 6. The first line intersects the circle in two places. The second line is tangent to the circle.

The points that satisfy both conditions are (2,5), (2,-3), and (6,1).

See the image below:

36. In the diagram below, BFCE, AB ⊥ BE, DE ⊥ BE, and ∠BFD ≅ ∠ECA.
Prove that △ABC ∼ △DEF.

Answer:

To prove that two triangles are similar, you have to show that two sets of angles are congruent. You are given one pair of angles and also that the pairs of sides are perpendicular, meaning that each triangle has a right angle. That's two angles, so you can use AA.

Your proof would go something like this:

Statement	Reason
1. AB ⊥ BE, DE ⊥ BE, ∠BFD ≅ ∠ECA	1. Given
2. Angle B is a right angle.	2. Definition of perpendicular.
3. Angle E is a right angle.	3. Definition of perpendicular.
4. ∠B ≅ ∠E	4. All right angles are congruent.
5. △ABC ∼ △DEFE	5. AA Theorem

37. In the diagram below of △ADE, B is a point on AE and C is a point on AD such that BC || ED, AC = x − 3, BE = 20, AB = 16, and AD = 2x + 2. Find the length of AC.

Answer:

The triangles are similar so the sides are proportional. AB/AC = AE/AD.

(16)/(x - 3) = (16 + 20) / (2x + 2)

16(2x + 2) = 36(x - 3)

32x + 32 = 36x - 108

140 = 4x

x = 35

AC = x - 3 = 35 - 3 = 32.

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Friday, April 22, 2022

Geometry Problems of the Day (Geometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2011

Part II: Each correct answer will receive 2 credits. Partial credit is possible.

32. In the diagram below of circle O, chord AB bisects chord CD at E. If AE = 8 and BE = 9, find the length of CE in simplest radical form.

Answer:

According to the Intersection Chords Theorem, the product (AE)(BE) = (CE)(DE). Since CD is bisected, then CE = DE.

So x² = (8)(9) = 72

Then x = √(72) = √(36 * 2) = 6√(2)

30. On the diagram below, use a compass and straightedge to construct the bisector of ∠ABC. [Leave all construction marks.]

Answer:

To bisect the angle, place the compass at point B. Draw an arc from BA to BC. From the points on the rays where the arc intercepted it, draw another arc. These two arcs will intersect each other. Mark a point and then draw the bisector from B through this point.

See the image below:

34. Find the slope of a line perpendicular to the line whose equation is 2y − 6x = 4.

Answer:

The slopeof the line perpendicular to the given line will have a slope that is the inverse reciprocal. That is, the two slopes will have a product of -1.

The equation in Standard Form is -6x + 2y = 4. The slope of that line is -A/B = -(-6)/2 = 3.

The slope of the perpendicular line would therefore be -1/3.

End of Part II.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Gnomon

(Click on the comic if you can't see the full image.)

I feel a sense of ignomony about this one...

This one has had a sticky note for a week waiting for me to look at it and say, "Oh, yeah, let me draw that."

ObMath: There's a parallelogram in the garden. If you remove the similar parallelogram with the gourds, then the L-shaped remainder is called the gnomon.

And it's filled with gnomes.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Thursday, April 21, 2022

Geometry Problems of the Day (Geometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2011

Part II: Each correct answer will receive 2 credits. Partial credit is possible.

29. In the diagram below of △ACD, B is a point on AC such that △ADB is an equilateral triangle, and △DBC is an isosceles triangle with DB ≅ BC. Find m∠C.

Answer:

Look at the image below:

All of the angles in the equilateral triangle are 60 degrees. Angles ABD and DBC are supplementary, so angle DBC is 120 degrees.

Angle DBC is also the vertex angle of the isosceles triangle making angles BDC and C the base angles, which must be congruent.

180 - 120 = 60 and 60 / 2 = 30, so m∠C = 30.

30. Triangle ABC has vertices A(−2,2), B(−1,−3), and C(4,0). Find the coordinates of the vertices of △A′B′C′, the image of △ABC after the transformation r_x-axis.
[The use of the grid below is optional.]

Answer:

A reflection across the x-axis would flip the sign of the y-coordinate. If the original is positive, then the image is negative and vice versa. A point on the x-axis will reflect onto itself. If this doesn't seem correct to you, then graph it.

A(−2,2) is reflected to A'(-2,-2).

B(−1,−3) is reflected to B'(-1,3).

C(4,0) is reflected onto itself, C'(4, 0).

31. Find, in degrees, the measures of both an interior angle and an exterior angle of a regular pentagon.

Answer:

A pentagon has five sides and angles. The total number of degrees in any pentagon is (5 - 2)*180 = 540.

In a regular pentagon, each angle is the same size, so 540 / 5 = 108 degrees for each interior angle.

The exterior angles are supplementary, so 180 - 108 = 72 degrees.

You could also do these in the opposite order.

The sum of the exterior angles of any convex polygon is 360 degrees. Therefore each individual exterior angle in a regular pentagon must be 360 / 5 = 72 degrees.

Each interior angle is supplementary to the exterior angle, so 180 - 72 = 108 degrees.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction!

If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.