Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, January 2011

Part I: Each correct answer will receive 2 credits.


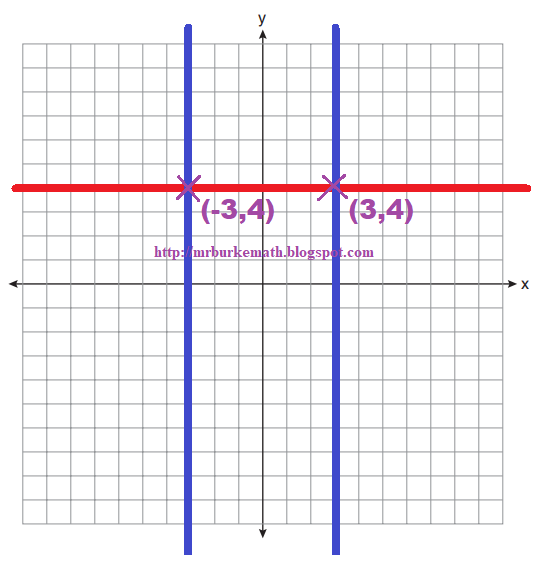
1. Which graph does not represent a function?

Answer: 4) [SEE IMAGE]

The last choice fails the vertical-line test, so it is not a function.

No x value can have multiple y values in a function.

2. The roots of the equation x² − 10 x + 25 = 0 are

1) imaginary
2) real and irrational
3) real, rational, and equal
4) real, rational, and unequal

Answer: 3) real, rational, and equal

If you graph the equation in your graphing calculator, you will see that it touches the x-axis at its vertex. That eliminates Choices (1) and (4).

If you check the Table of Values, you will see that the vertex is x = 5. You could also have used x = -b/(2a) to find that.

So the roots are rational. And they are equal.

If you factored, you would have had y = (x - 5)(x - 5), which tells you x = 5 is the only root.

3. Which values of x are solutions of the equation x³ + x² − 2x = 0?

1) 0, 1, 2
2) 0, 1, -2
3) 0, -1, 2
4) 0, -1, -2

Answer: 2) 0, 1, -2

Obviously x = 0 is a solution, and it's in all four choices. You can factor or you can use substitution to find the other two. Since the final sign is negative, you know it has to be (2) or (3), so if you are substituting, start with those.

x³ + x² − 2x = 0

x(x² + x − 2 = 0

x(x + 2)(x - 1) = 0

x = 0 or x = -2 or x = 1

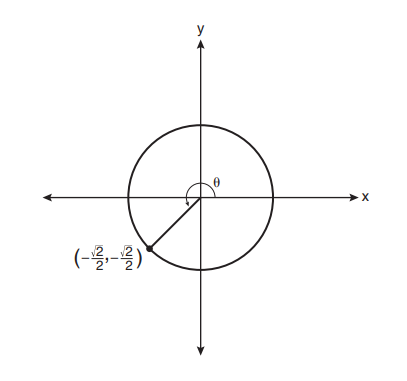
4. In the diagram below of a unit circle, the ordered pair (−√2/2, −√2/2) represents the point where the terminal side of θ intersects the unit circle.

What is m∠θ?

1) 45
2) 135
3) 225
4) 240

Answer: 3) 225

First, the point is in Quadrant III, so the measure must be 1800 < θ < 270. This eliminates Choices (1) and (2).

Second, you should know that (√2/2, √2/2) represents 45 degrees. Add 180 to that and you have 225, which is Choice (3).

5. What is the fifteenth term of the sequence 5, −10, 20, −40, 80, . . . ?

1) −163,840
2) −81,920
3) 81,920
4) 327,680

Answer: 3) 81,920

Notice that the odd terms are positive, so the fifteenth term (15th, not 50th) must be positive. Eliminate Choices (1) and (2).

The common ratio is the sequence is -10/5 = -2, 20/-10 = -2, etc. (You will notice that it does NOT have a common difference, so it is not arithmetic.)

The fifteenth term is (5)(-2)¹⁴ = 81,920.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2011

Part I: Each correct answer will receive 2 credits.


1. In the diagram below, AB, BC, and AC are tangents to circle O at points F, E, and D, respectively, AF = 6, CD = 5, and BE = 4.

What is the perimeter of △ABC?

1) 15
2) 25
3) 30
4) 60

Answer: 3) 30

When two tangents of a circle intersect, the segments between the point of intersection and the circle will be congruent. So AD ≅ AF; BE ≅ BF; CD ≅ CE.

So the perimeter is 6 + 6 + 5 + 5 + 4 + 4 = 30, or 2 * (6 + 5 + 4) = 30.

2. Quadrilateral MNOP is a trapezoid with MN || OP. If M′N′O′P′ is the image of MNOP after a reflection over the x-axis, which two sides of quadrilateral M′N′O′P′ are parallel?

1) M'N' and O'P'
2) M'N' and N'O'
3) P'M' and O'P'
4) P'M' and N'O'

Answer: 1) M'N' and O'P'

A reflection doesn't change the shape of the object. Lines that were parallel will still be parallel in the image.

If MN || OP in the pre-image, then M'N' || O'P' in the image.

3. In the diagram below of △ABC, D is the midpoint of AB, and E is the midpoint of BC.

If AC = 4x + 10, which expression represents DE?

1) x + 2.5
2) 2x + 5
3) 2x + 10
4) 8x + 20

Answer: 2) 2x + 5

The midsegment is half of the length of the side of the triangle that it is parallel to.

Half of 4x + 10 is 2x + 5.

4. Which statement is true about every parallelogram?

1) All four sides are congruent.
2) The interior angles are all congruent.
3) Two pairs of opposite sides are congruent.
4) The diagonals are perpendicular to each other.

Answer: 3) Two pairs of opposite sides are congruent

The opposite sides of a parallelogram are always congruent.

All four sides will be congruent only in a rhombus (including squares). The interior angles are all congruent only in rectangles (including squares). The diagonals are perpendicular only in rhombuses.

5. The diagram below shows a rectangular prism.
Which pair of edges are segments of lines that are coplanar ?

1) AB and DH
2) AE and DC
3) BC and EH
4) CG and EF

Answer: 3) BC and EH

The edges of a prism that are coplanar will be pointed in the same direction. If they aren't pointed in the same direction, they will be skew.

AB goes front to back, but DH goes up and down. Eliminate Choice (1).

AE goes up and down, but DC goes front to back. Eliminate Choice (2).

BC goes left to right, and EH goes left to right. This is the correct answer.

CG goes up and down, EF goes front to back. Eliminate Choice (4).

at long before the end.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Calendar Problems

As mentioned in other posts, I follow the "Math a Day" Calendar posts on Twitter, and I've even supplied the "official" answer a few times.

I like them so much that I starting using them as warmups in my own classes. The problem was that sometimes the question was at a level beyond my students' current abilities or they were just trivial in nature. Moreover, if an AP walked in, I'd think that they'd prefer to see a "Do Now" that related to what was being covered that day. To that extent, I started writing some of my own.

What follows are a handful of the calendar problems that I created. If any of Rebecca Rappaport's snuck through, allow me to apologize in advance for the error. The answers to each should be a whole number between 1 and 31. The ones below are not necessarily sequential.

If this is popular, I can make it a regular thing. Maybe every other Thursday or so, assuming I have enough to post (and I remember to schedule the post).

As always, math teachers are welcome to use these in their classrooms. If you put them online, please credit this blog. And a link would be nice. Maybe a comment saying "Howdy"? Please refrain from adding them from things that you intend to sell.

Enjoy!

Schrödinger and Hobson

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

Hobson's Choice was no choice at all. You go to class or you go home.

But you never know what kind of day it will be until you get there and start it.

And for newer readers, Moira was one of the first students to appear in (x, why?), and one of the few to actually age. Ken's daughter will eventually be another who starts aging.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Algebra Problems of the Day (Integrated Algebra Regents, January 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, January 2011

Part I: Each correct answer will receive 2 credits.


1. Given:

X = {1, 2, 3, 4}
Y = {2, 3, 4, 5}
Z = {3, 4, 5, 6}

What is the intersection of sets X, Y, and Z ?

1) {3, 4}
2) {2, 3, 4}
3) {3, 4, 5}
4) {1, 2, 3, 4, 5, 6}

Answer: 1) {3, 4}

The intersection of the three sets are the elements that can be found within all three sets.

Only 3 and 4 are in all three of the sets. This is Choice (1).

That is choice (3).

Choice (2) is the intersection of X and Y.

Choice (3) is the intersection of Y and Z.

Choices (4) is the union of X, Y, and Z, and contains all the elements that could be found in ANY of the three sets.

2. Which graph could be used to find the solution of the system of equations y = 2x + 6 and y = x² + 4x + 3?

Answer: 4) [See image]

The parabola opens upward (and has a y-intercept of 3) so eliminate Choices (2) and (3). The straight line has a slope of 2 and y-intercept of 6, so eliminate Choice (1).

Only Choice (4) remains.

3. What is the relationship between the independent and dependent variables in the scatter plot shown below?

1) undefined correlation
2) neagtive correlation
3) positive correlation
4) no correlation

Answer: 3) positive correlation

As x gets higher, y generally goes higher. That is a positive correlation.

There is no such thing as an undefined correlation.

4. Tim ate four more cookies than Alice. Bob ate twice as many cookies as Tim. If x represents the number of cookies Alice ate, which expression represents the number of cookies Bob ate?

1) 2 + (x + 4)
2) 2x + 4
3) 2(x + 4)
4) 4(x + 2)

Answer: 3) 2(x + 4)

T = A + 4 and B = 2T

So B = 2(A + 4). Using x instead of A, you have Choice (3).

5. 3) 2(x + 4)

1) {(3/4,0), (0,1), (3/4 ,2)}
2) {(-2,2), (-1/2,1), (-2,4)}
3) {(-1,4), (-0,5), (0,4)}
4) {(2,1), (4,3), (6,5)}

Answer: 4) {(2,1), (4,3), (6,5)}

In a function, every input can only have one output. The input cannot repeat with a different output number. (And since this is written as a set, it shouldn't repeat with the same output number either -- but that's set notation, not the definition of a function.)

The output can repeat with different inputs.

In Choice (1), x = 3/4 repeats. In Choice (2), x = -2 repeats. In Choice (3), x = 0 repeats.

Choice (4) has no repeats in the input.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2011

Part IV: A correct answer will receive 6 credits. Partial credit is available.


39. Solve the following system of equations algebraically:

5 = y − x

4x² = −17x + y + 4

Answer:

Solve the first equation for y and then substitute into the second equation. Or subtract the first equation from the second equation.

Substitution:

y = x + 5
4x² = −17x + x + 5 + 4
4x² = −16x + 9

Elimination:

4x² = −17x + y + 4
5 = - x + y
4x² - 5 = −16x + 4
4x² = −16x + 9

Continuing:

4x² + 16x - 9 = 0

(ac) = -36

Factors of -36 that = 16 are +18 and -2.

4x² - 2x + 18x - 9 = 0

2x(2x - 1) + 9(2x - 1) = 0

(2x + 9)(2x - 1) = 0

2x + 9 = 0 or 2x - 1 = 0

2x = -9 or 2x = 1

x = -9/2 or x = 1/2

For each x, find the matching y:

x = -9/2, 5 = y - (-9/2), y = 1/2, (-9/2, 1/2)

x = 1/2, 5 = y - (1/2), y = 11/2, (1/2, 11/2)

End of Exam

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2011

Part IV: A correct answer will receive 6 credits. Partial credit is available.


38. In the diagram below, PA and PB are tangent to circle O, OA and OB are radii, and OP intersects the circle at C.

Prove: ∠AOP ≅ ∠BOP

Answer:

You can prove the two angles are congruent by proving that the triangles are congruent. You know that the tangents make right angles, that the radii are congruent and that they share their hypotenuse. So by the HL Theorem, the two triangles are congruent.

You could also make use of the fact that the two tangents must be congruent, so you could use either SSS or SAS with the tangents, right angles, and radii.

Statement	Reason
1. PA and PB are tangent to circle O, OA and OB are radii, and OP intersects the circle at C	Given
2. ∠OAP is a right angle	Tangents intersect radii at right angles
3. ∠OBP is a right angle	Tangents intersect radii at right angles
4. ∠OAP ≅ ∠OBP	All right angles are congruent
5. OA ≅ OB	All radii in a circle are congruent
6. OP ≅ OP	Reflexive Property
7. Triangle OAP ≅ Triangle OBP	HL Theorem
8. ∠AOP ≅ ∠BOP	CPCTC

End of Part Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2011

Part III: Each correct answer will receive 4 credits. Partial credit is available.


36. Express as a single fraction the exact value of sin 75°.

Answer:

If you're one of those people who have memorized, sine and cosine for 15 and 75 degrees, then you know the answer, but they still want to see the work. You can use the formula for the Sum of Two Angles.

sin(A + B) = sin A cos B + cos A sin B

sin (45 + 30) = sin 45 cos 30 + cos 45 sin 30

sin (75) = (√(2)/2)(√(3)/2) + (√(2)/2)(1/2)

= (√(6)/4) + (√(2)/4)

= (√(6) + √(2)) / 4

37. Solve the inequality −3|6 − x| < −15 for x. Graph the solution on the line below.

Answer:

Divide both sides by -3 and flip the inequality. Then split the equation in two to solve the Absolute Value

−3|6 − x| < −15

|6 − x| > 5

6 - x < -5 or 6 - x > 5

-x &t -11 or -x > -1

x > 11 or x < 1

Your number should look like the one below:

38. The probability that a professional baseball player will get a hit is 1/3. Calculate the exact probability that he will get at least 3 hits in 5 attempts.

Answer:

The probability that he will get at least 3 hits is equal to the probability that he will get 3 plus the probability that he will get 4 plus the probability that he will get 5.

P(3) = ₅C₃(1/3)³(2/3)² = 10(4/243)

P(4) = ₅C₄(1/3)⁴(2/3) = 5(2/243)

P(5) = ₅C₅(1/3)⁵ = 1/243

P(3 or 4 or 5) = 51/243

End of Part III

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Parallel Universes

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

Why are these parallel worlds always evil? Maybe they have other things going on.

This was one of those times where I thought I could make a quick comic on a Friday. Right away, I knew that wouldn't be the case. In the early years of this comic, I refrained from having female characters for the simple reason that they didn't look right given how I drew the men -- a circle sitting on top of a parallelogram. Making that look female wasn't comical, it was sad. Now that I'm better at it (I won't say "good"), I thought I could modify my two main characters, which begs the question: what was I thinking? I knew that was a dangerous path to tread. But I went there.

I wouldn't hold out hope for seeing more of those two, unless there's overwhelming demand. And this blog doesn't get overwhelming demands.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2011

Part III: Each correct answer will receive 4 credits. Partial credit is available.


35. On the set of coordinate axes below, graph the locus of points that are equidistant from the lines y = 6 and y = 2 and also graph the locus of points that are 3 units from the y-axis. State the coordinates of all points that satisfy both conditions.

Answer:

The locus of points equidistant from horizontal lines y = 6 and y = 2 is the horizontal line y = 4, which is right in the middle of it. The locus of points 3 units from the y-axis is two vertical lines at x = 3 and x = -3.

The vertical lines intersect the horizontal line at (-3,4) and (3,4).

36. In the diagram below, tangent ML and secant MNK are drawn to circle O. The ratio mLN : mNK : mKL is 3:4:5. Find m∠LMK.

Answer:

If the circle is divided into three arcs, then those three arcs must add up to 360 degree. If they are in the ratio of 3:4:5, then the following is true;

3x + 4x + 5x = 360

12x = 360

x = 30

This means that mLN = 3(30) = 90, mNK = 4(30) = 120, and mKL = 5(30) = 150.

The measure of angle LMK is (mKL - mLN) / 2 = (150 - 90) / 2 = 60 / 2 = 30 degrees.

37. Solve the following system of equations graphically.

2x² − 4x = y + 1

x + y = 1

Answer:

Graph and label both lines. Label the points of intersection, and state the coordinates.

Rewrite the quadratic equation as y = 2x² - 4x - 1, and the linear equation as y = -x + 1.

The Axis of Symmetry of the parabola is x = -(-4)/(2(2)) = 1. When x = 1, y = 2(1)² - 4(1) - 1 = -3, so the vertex is (1,-3). You can start plotting points from there.

Your graph should look like the one below:

You may want to check on your calculator that 2(-1/2)² - 4(-1/2) = (3/2) + 1 because you can't really go by "looks".

2(-1/2)² - 4(-1/2) = 5/2

3/2 + 1 = 5/2

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones. The Integrated Algebra Regents covered most of the same material as the current Algebra Regents, with a few differences.

More Regents problems.

Integrated Algebra Regents, June 2011

Part IV: Each correct answer will receive 4 credits. Partial credit is available.


37. Solve algebraically for x:

3 / 4 = -(x + 11) / (4x) + 1 / (2x)

Answer:

You can multiply the entire equation by 4x to eliminate the fractions, or you can multiply the final fraction by (2/2), which would allow you to combine the fractions, which allows you to cross-multiply to solve the proportion.

Method 1:

(4x) [ 3 / 4 = -(x + 11) / (4x) + 1 / (2x) ]

3x = -(x + 11) + 2

3x = -x - 11 + 2

3x = -x - 9

4x = - 9

x = -9/4

Method 2:

3 / 4 = -(x + 11) / (4x) + [1 / (2x)][2/2]

3 / 4 = -(x + 11) / (4x) + 2 / (4x)

3 / 4 = (-x - 11) / (4x) + 2 / (4x)

3 / 4 = (-x - 9) / (4x)

12x = -4x - 36

16x = -36

x = -36/16 = -9/4

Note: only do one of the above. If you solve it twice, both will be graded and you will get the average of the two of them, not the better one.

38.An outfit Jennifer wears to school consists of a top, a bottom, and shoes. Possible choices are listed below.

Tops: T-shirt, blouse, sweater

Bottoms: jeans, skirt, capris

Shoes: flip-flops, sneakers

List the sample space or draw a tree diagram to represent all possible outfits consisting of one type of top, one type of bottom, and one pair of shoes.

Determine how many different outfits contain jeans and flip-flops.

Determine how many different outfits do not include a sweater.

Answer:

A sample space is a listing of ALL the possible combinations. A tree diagram should be smaller. Note that there are 3 * 3 * 2 = 18 possibilities. You can abbreviate, using just the first letter, except that you should use Sw for sweater, Sk for skirt, and Sn for sneakers to avoid confusion -- even if you think it's "obvious".

The sample space would be:

T J F, T J Sn, T Sk F, T Sk Sn, T C F, T C Sn

B J F, B J Sn, B Sk F, B Sk Sn, B C F, B C Sn

Sw J F, Sw J Sn, Sw Sk F, Sw Sk Sn, Sw C F, Sw C Sn

A tree diagram would look like this:

The number of outfits containing jeans and flip-flops is 3. You don't need to provide work IF you answered the first part of the problem. If you did NOT make a tree diagram or sample space then you MUST list the three outfits here to get the credit.

Twelve outfits do not include a sweater. Again, the tree diagram/sample space is the work for this question. If you didn't answer it, you need to explain how you got your answer, such as using the Counting Principle.

39. Solve the following system of inequalities graphically on the set of axes below.

3x + y < 7

y ≥ 2/3 x - 4

State the coordinates of a point in the solution set.

Answer:

Do NOT pick the intersection point or any point on the dashed line as a point in the solution. Nothing on the dashed line, including the intersection of the two lines, is a solution.

You can rewrite the first equation as y < -3x + 7 to put it in your graphing calculator.

Label at least one line (with the original inequality). Both is better.

Label any point in the double-shaded area.

You're graph should look like the one below. Point (0,0), for example, is a point in the solution set.

End of Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2011

Part II: Each correct answer will receive 2 credits. Partial credit is available.


32. If f(x) = x² − 6, find f⁻¹(x).

Answer:

Replace x with y, and replace f(x) with x. Then solve for y.

x = y² − 6

x + 6 = y²

y = + √(x + 6)

f⁻¹(x) = + √(x + 6)

33. Factor the expression 12t⁸ − 75t⁴ completely.

Answer:

Factor completely means that there will most likley be more than one step. You have a common factor is the coefficients, the same variable in both terms and a difference of squares to start with.

12t⁸ − 75t⁴

3t⁴(4t⁴ − 25)

3t⁴(2t² + 5)(2t² - 5)

You could also factor the last binomial again using √(2) and √(5), but that isn't necessary since 2 and 5 are not perfect squares.

34. Simplify the expression (3x^-4y⁵) / (2x³y^-7)^-2 and write the answer using only positive exponents.

Answer:

Since I'm typing this, I'm going to use the Rules for Exponents rather than playing with fractions:

(3x^-4y⁵) / (2x³y^-7)^-2

(3x^-4y⁵) / (4^-1x^-6y¹⁴)

(3x^{(-4 - -6)}y^{(5 - 14)}) / (4^-1)

(3x²y^-9) / (4^-1)

(3*4)x² / y⁹

12x² / y⁹

35. If f(x) = x² − 6 and g(x) = 2^x − 1, determine the value of (g ° f)(−3).

Answer:

Remember that (g ° f)(−3) means g(f(-3)), so you need to find f(-3) first.

f(-3) = (-3)² - 6 = 9 - 6 = 3

g(3) = 2³ - 1 = 8 - 1 = 7

(g ° f)(−3) = 7

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2011)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2011

Part II: Each correct answer will receive 2 credits. Partial credit is available.


32. A pentagon is drawn on the set of axes below. If the pentagon is reflected over the y-axis, determine if this transformation is an isometry.

Justify your answer. [The use of the set of axes below is optional.]

Answer:

An isometry doesn't change the figures size or shape. The orientation may change.

A reflection across the y-axis will change the orientation but not its size or shape. Therefore, this rigid transformation is an isometry.

33. In the diagram below of △ABC, D is a point on AB, E is a point on BC, AC || DE , CE = 25 inches, AD = 18 inches, and DB = 12 inches. Find, to the nearest tenth of an inch, the length of EB.

Answer:

The triangles are similar because the corresponding angles along the transversals of the parallel lines are congruent. That means the sides are proportional.

EB / DB = CB / AB

EB / 12 = (25 + EB) / (18 + 12)

30 EB = 12(25 + EB)

30 EB = 12 EB + 300

18 EB = 300

EB = 16.666...

EB is 16.7 inches.

34. In circle O, diameter RS has endpoints R(3a,2b − 1) and S(a − 6,4b + 5). Find the coordinates of point O, in terms of a and b. Express your answer in simplest form.

Answer:

Point O is the midpoint of diameter RS.

O( (3a + a - 6) / 2 , (2b - 1 + 4b + 5) / 2)

O ( (4a - 6) / 2 , (6b + 4) / 2)

O ( 2a - 3, 3b + 2)

End of Part II.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Rare Used Books

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

This is why editors like adjectives more than adverbs.

And nothings kills a family outing more than finding out that the place you wind up at isn't what you thought it was going to be.

We haven't seen Michele in a while.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.