Happy Halloween 2021!

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

Happy Halloween

Like many of my age and/or generation, the first real Halloween special on TV, and something that could be classified as "appointment television" was the annual showing of "It's the Great Pumpkin, Charlie Brown!" It's a pity that many younger people don't get the same joy from it's simplicity. The animation is old and program itself doesn't seem to get a lot of advance advertising on the networks. you have togo look for it, or find it on streaming. And, let's face it, it's a bit of a downer, which we'd watch again and again wondering if it would be happier the year after or not. (Keep of like watching reruns of Gilligan's Island for years to see if they'd ever get rescued.)

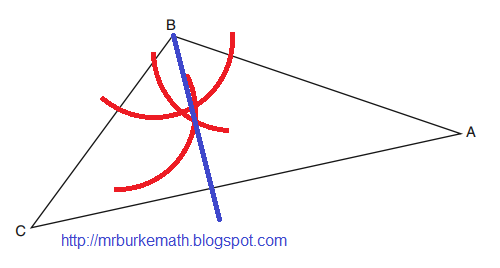
As for the math, a "great circle" is any circle around a sphere that has the center of the sphere as its center. A great arc would be a portion of that circle and would represent the shortest distance between two points on the outside of a sphere.

There may be a bonus Halloween comic tomorrow (Monday) because there was a school-based comic that was to appear on Friday, but between being busy with school and just being extremely tired on Saturday, it didn't get done. And if I save it for next year, it won't likely happen.

Happy Halloween, everyone!

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, January 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, January 2012

Part I: Each correct answer will receive 2 credits.


1. Line n intersects lines l and m, forming the angles shown in the diagram below.

Which value of x would prove l || m?

1) 2.5
2) 4.5
3) 6.25
4) 8.75

Answer: 2) 4.5

If the two lines are parallel, then the Altenate Interior Angles must be congruent. So write an equation that says that they equal each other:

18x - 12 = 6x + 42
12x = 54
x = 54/12 = 4.5

You also could have plugged in each choice into the two expressions to find which value made them equal.

2. In a given triangle, the point of intersection of the three medians is the same as the point of intersection of the three altitudes. Which classification of the triangle is correct?

1) scalene triangle
2) isosceles triangle
3) equilateral triangle
4) right isosceles triangle

Answer: 3) equilateral triangle

If choice (4) were correct, then Choice (2) would also be correct. So we can eliminate Choice (4). Also, the altitudes in a right triangle meet at the right angle. Medians don't meet at any of the vertices.

If the centroid and the orthocenter are the same point, then the triangle must be an equilateral triangle where each altitude is also a perpendicular bisector and an angle bisector.

3. A circle has the equation (x - 2)² + (y + 3)² = 36. What are the coordinates of its center and the length of its radius?

1) (-2,3) and 6
2) (2,-3) and 6
3) (-2,3) and 36
4) (2,-3) and 36

Answer: 2) (2,-3) and 6

The equation of a circle is given by the formula (x - h)² + (y - k)² = r², where (h,k) in the center of the circle and r is the radius. Note that there are MINUS signs in the formula, so the signs will be flipped.

If r² = 36, then the radius is 6, not 36. Eliminate Choices (3) and (4).

The signs are flipped, so the center if (2,-3), which is Choice (2).

4. In the diagram below, MATH is a rhombus with diagonals AH and MT.
If m∠HAM = 12, what is m∠AMT?
1) 12
2) 78
3) 84
4) 156

Answer: 2) 78

The diagonals of a rhombus for four right triangles. So in each triangle, the two acute angles are complementary, adding up to 90 degress.

So 90 - 12 = 78.

5. A line segment has endpoints (4,7) and (1,11). What is the length of the segment?

1) 5
2) 7
3) 16
4) 25

Answer: 1) 5

Use the distance formula or Pythagorean Theorem.

d = √( (4 - 1)² + (11 - 7)² ) = √( (3)² + (4)² )
= √(9 + 16) = √(25) = 5

If you know your Pythagorean Triples, you should have gotten that long before the end.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2/Trigonometry Regents, January 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, January 2012

Part I: Each correct answer will receive 2 credits.


1. The yearbook staff has designed a survey to learn student opinions computations. on how the yearbook could be improved for this year. If they want to distribute this survey to 100 students and obtain the most reliable data, they should survey

1) every third student sent to the office
2) every third student to enter the library
3) every third student to enter the gym for the basketball game
4) every third student arriving at school in the morning

Answer: 4) every third student arriving at school in the morning

Surveying the students entering the building in the morning is the most random of the four choices. Not many students would be send to the office. And certain subgroups of people would be overrepresented or underrepresented by standing outside the library or the gym (or the offiice, too, for that matter), so there would be more bias in the results.

2. What is the sum of the first 19 terms of the sequence 3, 10, 17, 24, 31, …?

1) 1188
2) 1197
3) 1254
4) 1292

Answer: 3) 1254

It's a short enough list that you might consider just adding the numbers in your calculator, but since the numbers are increasing by 7, if you make a mistake along the way, it'll through off your final answer.

The formula for adding a finite sequence is in the back of the booklet: S = n(a₁ + a_n) / 2. However, we need to know a₁₉.

Since the sequence has a common difference of 7, the explicit formula is a_n = 7(n - 1) + 3. So a₁₉ = 7(19 - 1) + 3 = 129.

Put that into the formula: S = (19)(3 + 129) / 2 = 1254

3. Which expression, when rounded to three decimal places, is equal to -1.155?

1) sec (5π/6)
2) tan(49°20')
3) sin (-3π/5)
4) csc(-118°)

Answer: 1) sec (5π/6)

Choice (2) is the first one you can immediately eliminate. It would be in Quadrant 1, so it has a positvie value. Choice (3) can be eliminated because sin cannot be less than -1. The other all have negative values. Make sure you are measuring radians or degrees, as stated.

Choice (1) = -1.1547.

Choice (2) = 1.1639. Note: 20 minutes is .33333 degrees.

Choice (3) = -0.9510.

Choice (4) = -1.1325.

4. If f(x) = 4x - x² and g(x) = 1/x, then (f ° g)(1/2) is equal to

1) 4/7
2) -2
3) 7/2
4) 4

Answer: 4) 4

Find f OF g OF 1/2.

g(1/2) = 1 / (1/2) = 2

f(2) = 4(2) - (2)² = 8 - 4 = 4.

5. A population of rabbits doubles every 60 days according to the formula P = 10(2)^(t/60), where P is the population of rabbits on day t. What is the value of t when the population is 320?

1) 240
2) 300
3) 660
4) 960

Answer: 2) 300

You can check each of the choices, or we can solve for t.

P = 10(2)^240/60 = 10(2)⁴ = 160

P = 10(2)^300/60 = 10(2)⁵ = 320. This is the answer.

Working backward:

320 = 10(2)^t/60
32 = (2)^t/60
2⁵ = (2)^t/60
5 = t/60
t = 300

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra Problems of the Day (Integrated Algebra Regents, January 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Integrated Algebra Regents, January 2012

Part I: Each correct answer will receive 2 credits.


1. Which expression is equivalent to 64 - x²?

1) (8 - x)(8 - x)
2) (8 - x)(8 + x)
3) (x - 8)(x - 8)
4) (x - 8)(x + 8)

Answer: 2) (8 - x)(8 + x)

The Difference of Two Perfect Squares factors into their conjugates.

Choice (1) is 64 - 16x + x²

Choice (3) is x² - 16x + 64

Choice (4) is x² - 64, which is the inverse of what we want.

2. Mr. Smith invested $2,500 in a savings account that earns 3% interest compounded annually. He made no additional deposits or withdrawals. Which expression can be used to determine the number of dollars in this account at the end of 4 years?

1) 2500(1 + 0.03)⁴
2) 2500(1 + 0.3)⁴
3) 2500(1 + 0.04)³
4) 2500(1 + 0.04)³

Answer: 1) 2500(1 + 0.03)⁴

The formula is A = P(1 + r)^t, where r is the rate as a decimal and t is the time in years. In this example, 3% means r = 0.03 and t = 4 years.

Choice (2) has 30% interest for 4 years, which is great if you can find it.

Choice (3) has 4% interest for 3 years.

Choice (4) has 40% interest for 3 years.

3. What is 2√(45) expressed in simplest radical form?

1) 3√(5)
2) 5√(5)
3) 6√(5)
4) 18√(5)

Answer: 3) 6√(5)

Factor 45 into 9 * 5. Since 9 is a perfect square, √(9) = 3, and 2 * 3 = 6. This leaves only the factor of 5 under the radical sign.

4. Which graph does not represent a function?

Answer: 3) (see image)

Choice (3) fails the Vertical Line Test. There are values of x that have two distinct y values for outputs. You cannot have that in a function.

For example, the points (1, 1) and (1, -1) are both on the graph in Choice (3).

Choice (4) may be a piecewise graph, but it is still a function.

5. Timmy bought a skateboard and two helmets for a total of d dollars. If each helmet cost h dollars, the cost of the skateboard could be represented by

1) 2dh
2) dh/2
3) d - 2h
4) d - h/2

Answer: 3) d - 2h

He spent d dollars for eaverything. He spent 2h for the two helmets (they were h each). Whatever is left over must be the cost of the skateboard. So subtract d - 2h to find the skateboard.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2012

Part IV: A correct answer will receive 6 credits. Partial credit is possible.


38. On the set of axes below, solve the system of equations graphically and state the coordinates of all points in the solution.

y = (x - 2)² - 3
2y + 16 = 4x

Answer:

I'm actually surprised that there wasn't a second part to this question.

There is a parabola in vertex form. And a linear equation not quite in either Standard nor Slope-Intercept form. They could have 2, 1 or 0 solutions. Always label the solutions according to your graph, even if your graph is (or you think may be) incorrect.

The quadratic equation can be put into your calculator as is and you can find the points to plot. Or you can plot the Vertex at (2,-3) and start graphing from there. If you realize that the y values will increase by +1, +3, +5, etc., then you know that the next few points are at (3,-2), (4,1), (5,6) and (6,13) which is off the top of the graph. Since the parabola has symmetry, there are also points at (1,-2), (0,1), (-1,6), and then off the top of the graph.

Rewrite the linear equation so you can enter it into your calculator:

2y + 16 = 4x
2y = 4x - 16
y = 2x - 8

You have a slope of 2 and a y-intercept of -8. You can plot points at (0,-8), (1,-6), (2,-4), etc.

Your graph should look like the one below. Remember to label which line is which, using the original equations, and label the solutions.

There is only one solution at (3,-2). The line is tangent to the parabola, but depending upon how well you sketch the parabola, it might look like it crosses it twice. It doesn't. If it didn't, you wouldn't be able to read the solutions from a graph. You would need to do it algebraically. If you need to convince yourself, your calculator will show you that there is only one intersection point, as will algebra.

End of Exam.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2 Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2012

Part IV: A correct answer will receive 6 credits. Partial credit is possible.


39. Solve algebraically for all values of x:

81^{x3 + 2x2} = 27^(5x/3)

Answer:

Both 27 and 81 are powers of 3, so each expression can be rewritten as a power of 3. Once you do that, then the two exponent expressions can be set equal to each other.

81^{x3 + 2x2} = 27^(5x/3)

(3⁴)^{x3 + 2x2} = (3³)^(5x/3)

(3)^{(4)(x3 + 2x2)} = (3)^(3)(5x/3)

(3)^{(4x3 + 8x2)} = (3)^(5x)

4x³ + 8x² = 5x

4x³ + 8x² - 5x = 0

(x)(4x² + 8x - 5) = 0

(x)(4x² + 10x - 2x - 5) = 0

(x) ( (2x)(2x + 5) - 1(2x + 5) ) = 0

(x) (2x - 1)(2x + 5) = 0

x = 0 or 2x - 1 = 0 or 2x + 5 = 0

x = 0 or x = 1/2 or x = -5/2

According to the rubric, writing "(x) (2x - 1)(2x + 5) = 0" was worth only 4 of the 6 points. I would You needed to complete those last two lines to get full credit. One mistake at the end would have gotten you 5 points instead of all 6.

End of Part Exam

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2 Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2012

Part III: Each correct answer will receive 4 credits. Partial credit is possible.


36. Express in the simplest form:

( (4 - x²) / (x² + 7x + 12) ) / ( (2x - 4)/ (x + 3) )

Answer:

First thing, split the fraction multiplying by the reciprocal of the denominator:

(4 - x²) / (x² + 7x + 12) * (x + 3) / (2x - 4)

After this, you can factor the quadratics and then cross out common factors.

((2 - x)(2 + x)) / ((x + 3)(x + 4)) * (x + 3) / (2(x - 2))

((2 - x)(2 + x)) / ((x + 3)(x + 4)) * (x + 3) / (2(x - 2))

Note that (2 - x) is equal to (-1)(x - 2):

((-1)(x - 2)(2 + x)) / (x + 4)) * 1 / (2(x - 2))

((-1)(2 + x)) / (x + 4)) * 1 / (2)

(-2 - x) / (2(x + 4))

37. 7 During a particular month, a local company surveyed all its employees to determine their travel times to work, in minutes. The data for all 15 employees are shown below.

25 55 40 65 29
45 59 35 25 37
52 30 8 40 55 Determine the number of employees whose travel time is within one standard deviation of the mean.

Answer:

Put the data in the calculator and perform One Variable Statistics to find the mean and the standard deviation.

The mean is 40 and the standard deviation is 14.8. This means that we want the number of employees whose travel to are in the range of (40 - 14.9) to (40 + 14.9), or 25.1 - 54.9.

Make sure you list the standard deviation and the range. If you make a mistake, you can still get partial credit for a consistent answer.

There are 8 employees in that range: 40, 29, 45, 35, 37, 52, 30, 40.

38. The measures of the angles between the resultant and two applied forces are 60° and 45°, and the magnitude of the resultant is 27 pounds. Find, to the nearest pound, the magnitude of each applied force.

Answer:

If you create a parallelogram with a diagonal of 27, then the two forces can be drawn as the sides of the parallelogram. The diagonal forms 60 and 45 degree angles. You now have two triangles. The third angle is 180 - 60 - 45 = 75 degrees. Now you can use the Law of Sines to find the length of the sides:

Sin 60 / a = Sin 75 / 27
a = 27 sin 60 / sin 75 = 24.2 = 24 pounts.

Sin 45 / b = Sin 75 / 27
b = 27 sin 45 / sin 75 = 19.7 = 20 pounts.

End of Part III

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2012

Part III: Each correct answer will receive 4 credits. Partial credit is possible.


35. Given: AD bisects BC at E, AB ⊥ BC, DC ⊥ BC.

Prove: AB ≅ DC

Answer:

To prove that AB ≅ DC, you first need to show that the two triangles are congruent. We know that both triangles have right angles and vertical angles and that E is a midpoint of BC. That means that you can use ASA to show the triangles are congruent.

Start with all the Given information. Your last line MUST be what you are proving.

Statement	Reason
1. AD bisects BC at E, AB ⊥ BC, DC ⊥ BC	1. Given
2. Angle BEA ≅ Angle CED	2. Vertical angles are congruent.
3. BE ≅ CE	3. Definition of midpoint.
4. Angle B ≅ = Angle C	4. All right angles are congruent.
5. Triangle ABE ≅ Triangle DCE	5. ASA
6. AB ≅ DC	6. CPCTC

36. TThe coordinates of trapezoid ABCD are A(-4,5), B(1,5), C(1,2), and D(-6,2). Trapezoid A"B"C"D" is the image after the composition r_x-axis ° r_{y = x} is performed on trapezoid ABCD. State the coordinates of trapezoid A"B"C"D".
[The use of the set of axes below is optional.

Answer:

In a composition of transformations, you work from right to left. You are doing a reflection over the x-axis OF THE IMAGE of the reflection over y = x.

Without using the grid, here are the rules for reflections:

Reflection over the line y = x: (x, y) --> (y, x)

Reflection over x-axis (x, y) --> (x, -y)

You could conceivably do this in one step. DON'T. Show the middle step.

A(-4,5), B(1,5), C(1,2), and D(-6,2)

A'(5,-4), B'(5,1), C'(2,1), and D'(2,-6)

A"(5,4), B"(5,-1), C"(2,-1), and D"(2,6)

37. In the diagram below of circle O, chords RT and QS intersect at M. Secant PTR and tangent PS are drawn to circle O. The length of RM is two more than the length of TM, QM = 2, SM = 12, and PT = 8.

Find the length of RT.
Find the length of PS.

Answer:

Rules you need to know: (RM)(MT) = (QM)(MS)
(PT)(PR) = (PS)²

We know that QM = 2 and SM = 12. We also know that RM = TM + 2. (Be careful -- I first read that as "two times" not "two more than"!)

So

(x)(x + 2) = (2)(12)
x² + 2x = 24
x² + 2x - 24 = 0
(x + 6)(x - 4) = 0
x + 6 = 0 or x - 4 = 0
x = -6 or x = 4
Discard the negative answer.

Since x = 4, the TM = 4 and RM = 4 + 2 = 6, so RT = 10

PT = 8 and RT = 10, so PR = 18. I used x, so let y = PS

y² = (8)(18)
y² = 144
y = 12

So PS = 12.

End of Part III.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Math Horror Movies: The Hungry Cardioid

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

At least, I didn't make a Cardioid B joke.

There's no "B" movie named "The Hungry Heart" that I'm aware of. I was originally going for a "Tell-Tale Heart" comic. However, when I started working on it, my homemade ink blot was telling me Pac-Man, so I went that route. But then I needed a new title because there wasn't anything "tell tale" about Pac-Man. Maybe next year for this.

No promises on Cardioid B though. How's that for scary?

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.

Geometry Problems of the Day (Geometry Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2012

Part II: Each correct answer will receive 2 credits. Partial credit is possible.


32. Using a compass and straightedge, construct the bisector of ∠CBA. [Leave all construction marks.]

Answer:

From ∠CBA, draw an arc that intercepts both BA and BC. Next, from each of those new points on BA and BC, make an arc the same size as the first one. Make a point where these last two arc intersect. Draw the angle bisector from B through this point.

If you used the wrong angle, you can still get one credit.

33. The cylindrical tank shown in the diagram below is to be painted. The tank is open at the top, and the bottom does not need to be painted. Only the outside needs to be painted. Each can of paint covers 600 square feet. How many cans of paint must be purchased to complete the job?

Answer:

You need to find the lateral area of the cylinder. Then divide that by 600 and round up.

Lateral Area = 2 π r h = (2)(3.141592)(12)(22) = 1658.76

Cans of paint = 1658.76 / 600 = 2.76... = 3 cans

In problems like this, you always need to round up because if you round down, you won't have enough paint.

34. On the set of axes below, graph the locus of points that are 4 units from the line x = 3 and the locus of points that are 5 units from the point (0,2). Label with an X all points that satisfy both conditions.

Answer:

The locus 4 units from x = 3 are two vertical lines, four units away on either side. The locus around (0, 2) is a circle with radius 5, with (0,2) as its center.. So angle 2 is 180 - 90 - 63 = 27 degrees.

The circle with intersect the left vertical line in two places. Since it has a radius of 5, it won't intersect the line x = 7 (which is the right vertical line).

End of Part II.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2 Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2012

Part II: Each correct answer will receive 2 credits. Partial credit is possible.


32. Find, to the nearest tenth, the radian measure of 216°.

Answer:

The rate of conversion is 180° = &pi radians, 216°, which is about 1/6th more that 180, should be just a little bit under 4. (Figure about 3.14 plus another .52 or so. This is meant to measure a reasonable answer, not to be an accurate result.)

216 * π / 180 = 3.769911... = 3.8 radians.

33. Find the third term in the recursive sequence a_k+1 = 2a_k - 1, where a₁ = 3.

Answer:

Start with a₁ and calculate the next two terms:

a₁ = 3

a₂ = 2(a₁) - 1 = 2(3) - 1 = 5

a₃ = 2(a₂) - 1 = 2(5) - 1 = 9

34. The two sides and included angle of a parallelogram are 18, 22, and 60°. Find its exact area in simplest form.

Answer:

The area of a parallelogram can be found using the formula A=a·b·sin(θ). (Use half of that for a triangle.)

A=a·b·sin(θ) = 18 * 22 * sin(60) = 396 * √(3) / 2 = 198 √(3)

35. Write an equation for the graph of the trigonometric function shown below.

Answer:

The graph starts at 0, so it's sin, not cos. The amplitude is 3, and it starts in the negative direction instead of positive. And the period is π instead of 2π, meaning that it has a frequency of 2.

So the equation for this graph is y = -3 sin (2x).

Leave any part of that out, including the y =, and you'll lose one point. Make two mistakes and you will earn no points, unless you state both the amplitude = 3 and the frequency is 2 (which is worth a point).

End of Part II

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Geometry Problems of the Day (Geometry Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Geometry Regents, June 2012

Part II: Each correct answer will receive 2 credits. Partial credit is possible.


29. Triangle TAP has coordinates T(-1,4), A(2,4), and P(2,0). On the set of axes below, graph and label triangle T'A'P', the image of triangle TAP after the translation (x,y) → (x - 5,y - 1).

Answer:

Graph and label TAP. Then plot T', A' and P' by moving 5 boxes to the left and 1 box down. Label them. Then draw triangle T'A'P'.

30. In the diagram below, L || m and QR ⊥ ST at R.

If m∠1 = 63, find m∠2.

Answer:

Using alternate interior angles, you wil have two of the three angles of a triangle and you can find the last one.

Angle QTR is 63 degrees. Angle QRT is 90 degrees. So angle 2 is 180 - 90 - 63 = 27 degrees.

31. Two lines are represented by the equations x + 2y = 4 and 4y - 2x = 12. Determine whether these lines are parallel, perpendicular, or neither. Justify your answer.

Answer:

Find the slope of the two lines.

In standard form, Ax + By = C, the slope of a line is -A/B.

The slope of the first line is -A/B = -1/2.

THe slope of the second line is -A/B = -(-2)/4 = 1/2.

The two lines are neither parallel nor perpendicular.

The slopes are not the same nor are they inverse reciprocals.

If you forgot the formula -A/B, then you could have rewritten the equations in slope-intercept form:

x + 2y = 4
2y = -x + 4
y = -1/2 x + 2

4y - 2x = 12
4y = 2x + 12
y = 1/2 x + 3

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Algebra 2 Problems of the Day (Algebra 2 Regents, June 2012)

Now that I'm caught up with the current New York State Regents exams, I'm revisiting some older ones.

More Regents problems.

Algebra 2/Trigonometry Regents, June 2012

Part II: Each correct answer will receive 2 credits. Partial credit is possible.


28. Determine the value of n in simplest form.

i¹³ + i¹⁸ + i³¹ + n = 0

Answer:

i² = -1 and i⁴ = 1, then i⁶ = -1 and i⁸ = 1, etc.

i¹³ + i¹⁸ + i³¹ + n = 0
(i¹²)(i) + i¹⁸ + (i³⁰)(i) + n = 0
(1)(i) + (-1) + (-1)(i) + n = 0
-1 + n = 0
n = 1

29. The formula for continuously compounded interest is A = Pe^rt, where A is the amount of money in the account, P is the initial investment, r is the interest rate, and t is the time in years.

Using the formula, determine, to the nearest dollar, the amount in the account after 8 years if $750 is invested at an annual rate of 3%.

Answer:

Set up the equation and use your calculator.

A = Pe^rt
A = (750)e^(0.03)(8) = 953.436863
A = $953

30. Express cos θ (sec θ - cos θ), in terms of sin θ.

Answer:

Trigometry identities that will be helpful: sec θ = 1 / cos θ, and sin² θ + cos² θ = 1.

cos θ (sec θ - cos θ)
= cos θ ((1 / cos θ) - cos θ)
= 1 - cos² θ
= sin² θ

31. A cup of soup is left on a countertop to cool. The table below gives the temperatures, in degrees Fahrenheit, of the soup recorded over a 10-minute period.

Write an exponential regression equation for the data, rounding all values to the nearest thousandth.

Answer:

Put the data in lists and run an exponential regression. You'll get the following equation:

y = 180.377(0.954)^x

This is one of the times where there is no real work to show because it is assumed that you did everything in the calculator. Likewise, there is pretty much no way that you could have gotten the correct answer without using the calculator.

Because of this, there are only a few ways to get 1 credit, instead of two: not rounding correctly or swapping the digits (transcription error), for sure; writing a linear regression equation; or writing a correct exponential expression, instead of an equation.

That is to say, "180.377(0.954)^x" is worth 1 credit, as is "y = 180.377(0.95)^x". However, "180.377(0.95)^x" by itself is worth 0 credits.

More to come. Comments and questions welcome.

More Regents problems.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Math Horror Ways: Seven Ways ...

(Click on the comic if you can't see the full image.)

(C)Copyright 2021, C. Burke. "AnthroNumerics" is a trademark of Christopher J. Burke and (x, why?).

It's to DivIdE for!

I have probably used all of these with the exception of b\a. The colon notation looked out at first, because I usually see it written in a proportion more than as just a single ratio, which is division.

The backward notation is useful for the distinction between "a divided by b" and "b divided into a". There are, of course, the same thing, but students get confused by the wording. I assume it's similar to the problem with "20 less than a number" being written as N - 20 and not 20 - N.

But everyone should have learned their "gazintas" at an early age.

I also write Fiction! You can now preorder Devilish And Divine, edited by John L. French and Danielle Ackley-McPhail, which contains (among many, many others) three stories by me, Christopher J. Burke about those above us and from down below. Preorder the softcover or ebook at Amazon. Also, check out In A Flash 2020, by Christopher J. Burke for 20 great flash fiction stories, perfectly sized for your train rides. Available in softcover or ebook at Amazon. If you enjoy it, please consider leaving a rating or review on Amazon or on Good Reads.

Come back often for more funny math and geeky comics.